The accuracy class of a measuring instrument is a generalized characteristic determined by the limits of permissible basic and additional errors, as well as other properties affecting accuracy, the values of which are established in the standards for certain types of measuring instruments. The accuracy class of measuring instruments characterizes their properties in terms of accuracy, but is not a direct indicator of the accuracy of measurements performed using these instruments.
In order to estimate in advance the error that a given measuring instrument will introduce into the result, normalized error values are used. They mean the maximum errors for a given type of measuring instrument.
The errors of individual measuring instruments of this type may be different, have systematic and random components that differ from each other, but in general the error of a given measuring device should not exceed the standardized value. The limits of the main error and influence coefficients are entered in the passport of each measuring device.
The main methods for normalizing permissible errors and designating accuracy classes of measuring instruments are established by GOST.
On the scale of the measuring device, the value of the accuracy class of the measuring device is marked in the form of a number indicating the normalized value of the error. Expressed as a percentage, it can have values of 6; 4; 2.5; 1.5; 1.0; 0.5; 0.2; 0.1; 0.05; 0.02; 0.01; 0.005; 0.002; 0.001, etc.
If the accuracy class value indicated on the scale is circled, for example 1.5, this means that the sensitivity error δ s = 1.5%. This is how the errors of scale converters (voltage dividers, measuring shunts, measuring current and voltage transformers, etc.) are normalized.
This means that for a given measuring device the sensitivity error δ s= dx/x is a constant value for any value of x. The relative error limit δ (x) is constant and for any value of x is simply equal to the value of δ s, and the absolute error of the measurement result is defined as dx = δ sx
For such measuring instruments, the boundaries of the operating range within which such an assessment is valid are always indicated.
If on the scale of a measuring device the accuracy class number is not underlined, for example 0.5, this means that the device is normalized by the reduced zero error δ o = 0.5%. For such devices, for any values of x, the absolute zero error limit is dx = d o = const, and δ o = d o / xn.
With a uniform or power scale of a measuring device and a zero mark at the edge of the scale or outside it, the upper limit of the measurement range is taken as xn. If the zero mark is in the middle of the scale, then xn is equal to the length of the measurement range, for example, for a milliammeter with a scale from -3 to +3 mA, xn = 3 - (-3) = 6 A.
However, it would be a gross mistake to assume that an ammeter of accuracy class 0.5 provides an error of ±0.5% in the measurement results over the entire measurement range. The value of the error δ o increases in inverse proportion to x, that is, the relative error δ (x) is equal to the accuracy class of the measuring device only at the last mark of the scale (at x = xk). At x = 0.1xk it is 10 times greater than the accuracy class. As x approaches zero, δ (x) tends to infinity, that is, it is unacceptable to take measurements with such devices in the initial part of the scale.
On measuring instruments with a sharply uneven scale (for example, on ohmmeters), the accuracy class is indicated in fractions of the scale length and is designated as 1.5 with the “angle” sign below the numbers.
If the designation of the accuracy class on the scale of the measuring device is given in the form of a fraction (for example, 0.02/0.01), this indicates that the reduced error at the end of the measurement range is δ prk = ±0.02%, and at the zero of the range δ prk = -0.01%. Such measuring instruments include high-precision digital voltmeters, DC potentiometers and other high-precision instruments. In this case
where xk is the upper limit of measurement (the final value of the instrument scale), x is the measured value.
Source
Accuracy class
During laboratory measurements, it is necessary to know the accuracy of the measuring instruments, which in turn have certain characteristics and differ in design. Each of the measuring instruments (MI) has certain inaccuracies, which are divided into basic and additional. Situations often arise when it is not possible or simply not required to make a detailed calculation. Each measuring instrument is assigned a certain accuracy class, knowing which, you can find out its range of deviations.
Read also: Why you need to overclock your processor ⋆
Normalized error values will help you find out the errors of the measuring instrument in a timely manner. This definition should be understood as the limiting indicators for a measuring instrument. They can be different in size and depend on different conditions, but they should not be neglected in any case, because this can lead to a serious mistake in the future. The normalized values should be less than what the device shows. The limits of permissible error values and the necessary coefficients are entered in the passport of each dimensional measuring device. You can find out detailed standardization values for any device using the corresponding GOST.
Accuracy class
During laboratory measurements, it is necessary to know the accuracy of the measuring instruments, which in turn have certain characteristics and differ in design. Each of the measuring instruments (MI) has certain inaccuracies, which are divided into basic and additional. Situations often arise when it is not possible or simply not required to make a detailed calculation. Each measuring instrument is assigned a certain accuracy class, knowing which, you can find out its range of deviations.
Normalized error values will help you find out the errors of the measuring instrument in a timely manner. This definition should be understood as the limiting indicators for a measuring instrument. They can be different in size and depend on different conditions, but they should not be neglected in any case, because this can lead to a serious mistake in the future. The normalized values should be less than what the device shows. The limits of permissible error values and the necessary coefficients are entered in the passport of each dimensional measuring device. You can find out detailed standardization values for any device using the corresponding GOST.
Non-certified scales
If the scales are not certified, this does not mean that they are bad or of poor quality. The issue of counterfeit, defective and low-grade equipment is not raised at all in this article.
Certification of weighing equipment is a consequence, and not a source, of a certain level of technical characteristics of a metrological device. That is, the certificate is only a confirming legal document.
A measuring instrument may not be certified for various reasons:
- The foreign manufacturing company does not consider the Russian market as a priority. At the same time, their weighing equipment can be fully certified in other countries - China, Korea, the European Union, and the USA.
reference Information
If the scales are not certified only in Russia, the passport of the weighing equipment may indicate that the device has a certificate of approval of the type of measuring instrument according to the manufacturer’s technical documentation or something like that. This means that the equipment has documents and certificates that are not valid in the Russian Federation.
- The manufacturer is aimed at those areas of activity in which certification is not required. This reduces the cost of production, since certification costs significant funds.
- The scales were certified, but the certificate expired and was not renewed for some reason.
- The new device model is currently being certified. The certification period can take up to a year, and the products can already be offered to customers as a technological measuring instrument.
- A separate point can be made to high-precision microbalances manufactured abroad, which are not technically possible to certify in Russia - there is no equipment and technical verification regulations have not been developed. Such microbalances may be certified to one level of accuracy, but actually have another. That is, the weighing device is not certified for the entire range of errors that it can provide. Therefore, there are scales that are verified to read 0.001 mg, but actually have a scale division value of 0.0001 mg.
Uncertified scales are full-fledged measuring instruments; they simply cannot be used in some areas of activity that are regulated by the state. In most cases, their accuracy is identical to that of Class III-Medium scales.
What is the accuracy class of the device?
An accuracy class is a characteristic of a device, which is determined by the limits of the permissible main and additional errors, as well as other properties provided for by the standards for this type of product that affect the accuracy. This parameter is present in the technical characteristics of many devices that have reference output parameters, be they electronic or mechanical measuring devices. The accuracy class is the main characteristic of measuring equipment: scales, multimeters, oscilloscopes, instrumentation equipment and others. The higher this value for a device, the more such a device costs, this is due to the complexity of producing such products.
Normalized error
Characteristics and application of electronic scales I-Special accuracy class
Scales of the I-Special accuracy class are the most high-tech type of weighing equipment, and models of this class are the calling card of the manufacturer, showing its technological potential. Therefore, their error is minimized as much as production technology allows.
Scales of the I-Special class include particularly precise scales that are used for scientific research or for medical purposes. All of them, without exception, are analytical or laboratory.
Separately, we can distinguish microbalances and ultramicrobalances, the accuracy of which, even at the I-Special class level, is very high. But technically they still belong to the I-Medium accuracy class.
Discreteness values of scales of the I-Special accuracy class with different NEL
| NPV | Discreteness | NmPV | Typical types of scales |
| 2 g | 0.1 or 1 mcg | 0.05…1 mg |
|
| 5 g | 0.1 or 1 mcg | 0.05…2 mg | |
| 6 g | 0.1 or 1 mcg | 0.05…2 mg | |
| 10 g | 0.001 mg | 0.1…2.5 mg | |
| 11 g | 0.001 mg | 0.1…2.5 mg | |
| 20 g | 0.001 or 0.01 mg | 0.1…2.5 mg | |
| 22 g | 0.001 or 0.01 mg | 0.1…2.5 mg | |
| 30 g | 0.001 or 0.01 mg | 0.4…4 mg |
|
| 50 g | 0.01 mg or 0.1 mg | 1…10 mg | |
| 60 g | 0.01 mg or 0.1 mg | 1…10 mg | |
| 80 g | 0.01 or 0.1 mg | 1…10 mg | |
| 100 g | 0.01 or 0.1 mg | 1…10 mg | |
| 120 g | 0.01 or 0.1 mg | 1…10 mg | |
| 150 g | 0.01 or 0.1 mg | 1…10 mg | |
| 200 g | 0.01 or 0.1 mg | 1 mg...10 mg | |
| 250 g | 0.01 or 0.1 mg | 1 mg...10 mg | |
| 300 g | 0.1 mg | 10 mg | |
| 400 g | 0.1 mg | 10 mg | |
| 500 g | 0.1 mg | 10 mg | |
| 600 g | 1 mg | 0.1 g | |
| 800 g | 1 or 10 mg | 0.1…1 g | |
| 1 kg | 1 or 10 mg | 0.1…1 g | |
| 1.1 kg | 1 or 10 mg | 0.1…1 g | |
| 1.2 kg | 1 or 10 mg | 0.1 g…1 g | |
| 1.5 kg | 1 or 10 mg | 0.1 g…1 g | |
| 2 kg | 1 or 10 mg | 0.1…1 g | |
| 6 kg | 0.01 g | 1 g | |
| 8 kg | 0.01 g | 1 g | |
| 10 kg | 0.01 or 0.1 g | 1…10 g |
Attention!
The contents of the table are for informational purposes only and are not a complete list of all types of equipment and their characteristics.
Types of marking
The accuracy classes of absolutely all measuring instruments are subject to marking on the scale of these same instruments in the form of a number. Arabic numerals are used to indicate the percentage of normalized error. The designation of the accuracy class in a circle, for example the number 1.0, indicates that the error in the device’s needle readings will be equal to 1%.
If the designation uses a tick in addition to the number, this means that the scale length is used as a normalizing value.
Latin letters for designation are used if it is determined by the limits of absolute error.
There are devices on the scales of which there is no information about the accuracy class. In such cases, the absolute should be equated to one half of the smallest division.
Types of measurements
Measurement error is the amount of deviation from the true value of the measured indicators and quantities. The following formulas are used for calculation:
- Absolute error: Δ = Xd – Xmeas. It is calculated by subtracting the measured value from the real number. Expressed in units of measured indicators.
- Relative error: δ = (Δ ⁄ Xd)*100. Shows the percentage of deviation by absolute value and is calculated by the ratio of absolute to real, multiplied by 100%.
- Reduced error: γ = (Δ ⁄ Xн)*100. It is calculated based on the standard value, which allows you to specify the measurement range. In this case, the absolute value is divided by the normalizing value multiplied by 100%.
If there is a set of devices, then the overall characteristic is determined. First, the errors are brought to a single form, and then added up.
Limits
As mentioned earlier, the measuring device, thanks to standardization, already contains random and systematic errors. But it is worth remembering that they depend on the measurement method, conditions and other factors. In order for the value of the quantity to be measured to be 99% accurate, the measuring instrument must have minimal inaccuracy. The relative one should be about a third or a quarter less than the measurement error.
Weighing limits
This is the characteristic that raises the least number of questions from the consumer.
The upper (largest) weighing limit (ULL, Max) is the maximum load (weight) value that can be displayed on the scale display. If the sample mass is greater than this value, then the measurement results will not be accurate.
The lower (smallest) weighing limit (NmPV, Min) is the amount of mass below which the measurement error will be excessive. In other words, the scale display will not show any values.
The LEL should not be confused with the maximum load (Lim). If the mass of the sample is greater than the LEL, the measurement result will not be accurate. And if the mass is greater than Lim, then the device will break.
A Question of Choice
To install an electric meter in a private house or apartment, models that have a class of at least 2 are suitable.
In addition, when going to the store to buy an electric meter, you should know exactly the following characteristics:
- Phase of the electrical network. If the electrical network connected to the meter is single-phase, then the device must also be for a single-phase network. A three-phase electric meter can also be installed to track electricity usage, but such devices tend to be more expensive. When the meter is installed to measure three-phase current, the corresponding inscription must be indicated on it. Single-phase devices are not used to calculate three-phase current.
- The load under which this device will be operated. Depending on the maximum load that will be connected to the electricity metering device, a model is selected, on the body of which this indicator is indicated. For a standard load used in a private home, electric meter models designed for a maximum current of 60 A are used. If you plan to connect powerful electric heating boilers, then an electric meter is selected with an indicator of at least 100 A.
- If the electricity supplier can sell electricity at 2 tariffs, then the tariff of the meter is also taken into account when purchasing. Two-tariff devices allow you to save significantly on electricity bills. When using electricity at night, such a meter will record consumption separately. If the electricity supplier allows such payment, then installing a multi-tariff meter will allow you to use electricity more efficiently.
- Mounting method. Allows you to install the device in an existing box, or in place of a device that was previously installed.
Discreteness (division price)
Discreteness is a property of measurements that is the opposite of continuity. This is an indicator that varies between 2 adjacent divisions of weighing equipment (stable states). Hence the name – division price (denoted by “d”).
The division value is one of the key characteristics of standards for laboratory balances. The smaller it is, the higher the accuracy of weight measurement. For example, if you put a 3 kg weight on a scale with a resolution of 5 g, then the display will show a result of 3 kg. If you further add a 3.5g weight to the platform, the scales will show the result of weighing 3 kg and 5g. This is caused by the discreteness of the equipment.
ERRORS AND ACCURACY CLASSES OF ELECTRICAL INSTRUMENTS
ERRORS AND ACCURACY CLASSES OF ELECTRICAL INSTRUMENTS
The value measured by a device always differs from the true value by a certain number, called the device error. The errors of measuring instruments are determined by verification, i.e., by comparing the readings of the device being verified with the readings of a more accurate, standard device when they measure the same value. The value of the measured quantity, determined using a reference device, is considered to be valid. However, the actual value differs from the true one by the error inherent in this reference device. There are absolute, relative and reduced measurement errors.
ACCURACY OF MEASUREMENT INSTRUMENTS
The quality of a measuring device is characterized by its accuracy, which is assessed by the measurement error.
From the consideration of the above, it follows that impeccably accurate measurement of electrical quantities is technically impossible, i.e. the true value of the measured quantity cannot be determined using a measuring instrument. Therefore, the actual value of the measured quantity is taken as the true value.
The difference between the value of the quantity measured using the working device a
,, and its true value
a
is called the absolute measurement error:
The smaller the absolute error in comparison with the measured value, the higher the quality of the measurement. To characterize the measurement quality, the relative measurement error is introduced:
Since the magnitudes ai ah
differ little from each other, then often instead of
a
we substitute the value
a„
obtained directly from experience. The value of the absolute measurement error is influenced mainly by the error in readings, imperfection of measurement methods and the error of the instruments themselves.
Errors of electrical measuring instruments are divided into basic and additional. The main errors characterize the quality of the device itself; additional errors are caused by deviations of operating conditions from normal ones. The ratio of the largest value of the basic absolute error to the upper limit of measurement of the device determines the quality of the device itself. This ratio is called the reduced error. The given error is usually expressed as a percentage, and according to the value of the given error, all devices are divided into 8 accuracy classes: 0.05; 0.1; 0.2; 0.5; 1; 1.5; 2.5; 4.0. Devices that have a reduced error of more than 4% are considered extracurricular (these are panel and educational devices). However, the accuracy class of the device does not determine the accuracy of the measurement itself. To prove this position in the case where the absolute error does not depend on a
, multiply and divide the expression for the relative error by the upper limit of measurement am:
How to determine the accuracy class of an electrical measuring instrument, calculation formulas
To determine the accuracy class, you need to look at its case or user manual, in it you can see a number circled, for example , ① this means that your device measures a value with a relative error of ±1%.
But what to do if the relative error is known and it is necessary to calculate the accuracy class of, for example, an ammeter, voltmeter, etc. Let's look at the example of an ammeter: known ∆x=basic (absolute) error 0.025 (see instructions), number of divisions x=12
We find the relative error:
Y= 100×0.025/12=0.208 or 2.08%
( conclusion: accuracy class – 2.5).
It should be noted that the error is uneven over the entire scale range; by measuring a small value you can get the greatest inaccuracy and as the desired value increases, it decreases; for example, consider the following option:
Voltmeter with class p=±2, upper limit of device readings Xn=80V, number of divisions x=12
Absolute permissible error limit:
Relative error of one division:
If you need to perform a more detailed calculation, see GOST 8.401-80 clause 3.2.6.
V. Accuracy of measuring instruments.
The accuracy of a measuring device is its property that characterizes the degree of approximation of the readings of a given measuring device to the actual values of the measured value and is determined by the smallest value that can be determined reliably using this device.
The accuracy of the device depends on the value of the smallest division of its scale and is indicated either on the device itself or in the factory instructions (passport). Note that the measurement accuracy
is inversely proportional to the relative measurement error E: = .
The error of electrical measuring instruments is determined by the accuracy class (or reduced error Epr), which is indicated on the front side of the device with the corresponding number in a circle. The accuracy class of an instrument K is the ratio of the absolute error expressed as a percentage
to the limiting (nominal) value of the xpr of the measured value, i.e. to its greatest value that can be measured on the instrument scale (measurement limit):.
Knowing the accuracy class and measurement limit of the device, you can calculate its absolute error:
.
This error is the same for any measurement made using this device. Seven accuracy classes: 0.1; 0.2; 0.5; 1.0; 1.5; 2.5; 4.0. Devices of the first three accuracy classes (0.1; 0.2; 0.5) are called precision
and are used for precise scientific measurements, instruments of other accuracy classes are called
technical
. Devices without indicating the accuracy class are considered extracurricular.
Example . The current strength is measured in the circuit with an ammeter, the accuracy class of which is K = 0.5, and the scale has a measurement limit Ipr = 10 A. We find the absolute error of the ammeter:
It follows that the ammeter allows you to measure current with an accuracy of no more than 0.05 A, and therefore it is inappropriate to make a reading on the instrument scale with greater accuracy.
Let's assume that three current values were measured using this ammeter: I1=2 A; I2=5 A; I3=8 A. We find the relative error for each case:
; .
From this example it follows that in the third case the relative error is the smallest, that is, the larger the reading value on the device, the smaller the relative measurement error. That is why, for optimal use of instruments, it is recommended to select them so that the value of the measured value is at the end of the instrument scale. In this case, the relative error approaches the accuracy class of the device. If the accuracy of the device is unknown, then the absolute error is taken equal to half the value of the smallest division (ruler, thermometer, stopwatch). For calipers and micrometers – the accuracy of their verniers (0.1 mm, 0.01 mm).
Notes: 1) When making readings, make sure that the line of sight is perpendicular to the scale. To eliminate the so-called parallax error, many devices are equipped with a mirror (“mirror devices”). The experimenter's eye is positioned correctly if the instrument needle covers its image in the mirror.
2) For indirect measurements (for example, determining the volume of a cylinder by its diameter and height), all measured vertices should be determined with approximately the same relative accuracy.
3) When processing measurement results, it should be remembered that the accuracy of calculations must be consistent with the accuracy of the measurements themselves. Calculations performed with more decimal places than necessary result in a lot of unnecessary work. For example, if at least one of the quantities in any expression is defined with an accuracy of two significant figures, then there is no point in calculating the result with an accuracy of more than two significant figures. At the same time, in intermediate calculations it is recommended to save one extra digit, which later - when recording the final result - will be discarded. In the theory of errors, there is the following exception to the existing rounding rules: when rounding errors, the last digit retained is increased by one if the highest discarded digit is 3 or greater than 3.
4) Examples of final recording of measurement results:
Rationing
Accuracy classes of measuring instruments tell us information about the accuracy of such instruments, but at the same time it does not show the accuracy of the measurement performed using this measuring device. In order to identify in advance the error in the instrument readings, which it will indicate during measurement, people normalize the errors. To do this, they use already known normalized values.
Rationing is carried out according to:
- absolute;
- relative;
- given.
Formulas for calculating absolute error according to GOST 8.401
Each device from a specific group of devices for measuring dimensions has a certain value of inaccuracies. It may differ slightly from the established standardized indicator, but not exceed the general indicators. Each such unit has a passport in which the minimum and maximum error values are recorded, as well as coefficients that have an impact in certain situations.
Download GOST 8.401-80
All methods of standardizing measuring instruments and designating their accuracy classes are established in the relevant GOSTs.
Regulatory documents
There are several regulatory and technical documents that regulate the concept of accuracy and error class. The first document is GOST 13600-68, which establishes general provisions for the division of measuring instruments, as well as possible options for standardizing metrological indicators. The standard does not regulate technical issues for each individual device; instructions must apply to them.
CT issues are also presented in GOST 8.401-80 “SI accuracy classes general requirements”. This is the main document for the metrological service, which verifies measuring instruments. The document leads to uniformity of characteristics of measuring instruments, which makes it possible to compare values and determine the complex error of measurement systems.
Source
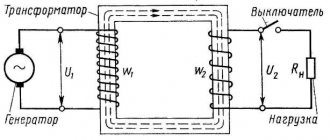
What are they used for?
Various types of instrument transformers are found both in small matchbox-sized devices and in large power installations. Their main purpose is to reduce primary currents and voltages to the values required for measuring devices, protective relays and automation. The use of step-down coils provides protection for the lower and higher rank circuits, since they are separated from each other.
Reducing agents are divided according to characteristics of operation and are intended for:
- measurements. They transmit secondary current to devices;
- protection of current circuits;
- applications in laboratories. Such reducing agents have a high accuracy rating;
- repeated conversion, they belong to intermediate instruments.
Measurement
An instrument transformer is necessary to reduce the high current of the main voltage and transfer it to measuring devices. Connecting standard devices to a high-voltage network would require cumbersome installations. It is neither economically profitable nor advisable to sell instruments of this size.
The use of step-down transformers allows the use of conventional measuring devices in normal mode, which expands the range of their applications. Due to the reduced voltage, they do not require additional modifications. The transformer separates the high-voltage network voltage from the supply voltage of the devices, ensuring safety from use. The accuracy of electrical energy metering depends on their class.
Protection
In addition to powering measuring instruments, step-down transformers supply voltage to protection and automatic blocking systems. Because voltage drops and surges occur in the network electrical network, which is detrimental to high-precision circuit equipment.
In power plants, equipment is divided into power and secondary, which controls the processes of the primary device connection circuit. High-voltage equipment is located in open areas or devices. Secondary equipment is located on relay strips inside distribution cabinets.
The intermediate element for transmitting information between power units and measuring, control, monitoring and protection devices are step-down or instrument transformers. They separate the primary and secondary circuits from the harmful effects of power units on sensitive measuring instruments, and also protect operating personnel from damage.
Classification of the meter by phase
Depending on what type of electrical network is installed in the house (single-phase or three-phase), you must purchase the appropriate meter:
- Single-phase metering device - installed in a single-phase (two-wire) network with a voltage of 220 V. Such electrical networks are mainly installed in apartments, individual residential buildings, small shops, and offices.
- Three-phase metering device - installed in a three-phase network with a voltage of 380 V or more. Such electrical networks are installed in large cottages, industrial facilities, large stores, restaurants, administrative buildings and warehouses, in a word - in large facilities.
Types of ammeters
They can be electromechanical or analogue, digital or electronic. The basic set usually consists of a detector, a transmitter and an indicator, a recorder or a storage device.
Analog devices are the oldest instruments in use. Although they are reliable for static and stable measurements, they are not suitable for dynamic and transient conditions. In addition, they are quite bulky and have limitations due to the use of a dial indicator.
Electronic instruments respond faster and are able to instantly detect dynamic changes in current in the network. An example is a digital multimeter, which is capable of measuring dynamic or transient current values in seconds.
How to determine the accuracy class of a pressure gauge
A pressure gauge is a measuring device that allows you to determine the value of excess pressure acting in a pipeline or in the working parts of various types of equipment.
Such devices are widely used in heating systems, water supply, gas supply, and other utility networks for municipal and industrial purposes. Depending on the operating conditions of the meter, there are certain restrictions on the permissible limit of its error. Therefore, it is important to know how to determine the accuracy class of a pressure gauge.
Classification of metal-cutting machines - all about equipment for metal processing
Metal-cutting machines produced by domestic manufacturers are divided into several categories, which are characterized by the corresponding classification. You can determine which category this or that equipment belongs to by its labeling, which says a lot to those who understand it. However, no matter what category the metal-cutting device belongs to, the essence of processing on it comes down to the fact that the cutting tool and the part perform form-building movements, and it is they that determine the configuration and dimensions of the finished product.
The most common types of metal-cutting machines: 1-6 - lathes, 7-10 - drilling, 11-14 - milling, 15-17 - planing, 18-19 - broaching, 20-24 - grinding.
Types of metal-cutting equipment
Depending on their purpose, metal-cutting machines are divided into nine main groups. These include the following devices:
- lathes - all types of lathe machines (in the marking they are indicated by the number “1”);
- drilling and boring - machines for performing drilling operations and boring (group “2”);
- grinding, polishing, finishing - metal-cutting machines for performing finishing, grinding, sharpening and polishing technological operations (group “3”);
- combined - metal-cutting devices for special purposes (group “4”);
- thread and gear processing - machines for processing elements of threaded and gear connections (group “5”);
- milling - machines for performing milling work (group “6”);
- slotting, planing and broaching - metal-cutting machines of various modifications, respectively, for planing, slotting and broaching (group “7”);
- cutting - equipment for performing cutting work, including saws (group “8”);
- different - examples of such metal-cutting units are centerless-grinding, saw-cutting and others (group “9”).
Groups and types of metal-cutting machines (click to enlarge)
In addition, metal cutting machines can be one of the following types:
- multi- and single-spindle, specialized (semi-automatic and automatic), multi-cutting copying, turret, drilling and cutting, rotary, frontal and special types of lathes;
- equipment for performing technological operations of boring and drilling: multi- and single-spindle, semi-automatic, drilling machines of vertical, horizontal and radial type, boring devices of coordinate, diamond and horizontal type, various drilling models;
- various types of grinding machines (flat, internal and cylindrical grinding), roughing and polishing equipment, sharpening and specialized units;
- types of metalworking machines designed for processing elements of gear and threaded connections: gear cutting machines (including those intended for processing conical wheels), gear cutting machines - for cylindrical gears, gear milling, thread cutting, thread and gear grinding, gear finishing, checking, thread milling, devices for processing the ends of teeth and elements of worm pairs;
- metal-cutting machines belonging to the milling group: cantilever (vertical, horizontal and universal models) and non-cantilever (vertical devices, longitudinal, copying and engraving models);
- planing equipment and models for similar purposes: longitudinal machines on which one or two stands are installed; horizontal and vertical broaching devices;
- cutting equipment: equipped with an abrasive wheel or a smooth metal disk, cutter or saws of various designs (band, disk, hacksaw); straightening-cutting types of metalworking machines;
- other types of machines for processing metal workpieces: dividing machines, used for monitoring drills and grinding wheels, filing, balancing, leveling and centerless roughing, sawing.
Vertical milling machine is one of the representatives of an extensive milling group
Classification of metal-cutting machines is also carried out according to the following parameters:
- by weight and overall dimensions of the equipment: large, heavy and unique;
- by level of specialization: machines designed for processing workpieces of the same size - special; for parts with different but similar sizes - specialized ones; universal devices that can be used to process parts of any size and shape;
- according to the degree of processing accuracy: increased - P, normal - N, high - B, especially high accuracy - A; There are also machines that can perform particularly precise processing - C, they are also called precision.
Machine markings
The classification of equipment intended for processing metal workpieces assumes that, having seen its markings, any specialist will immediately be able to tell which metal-cutting machine is in front of him. This marking contains alphabetic and numerical symbols that indicate individual characteristics of the device.
The first number is the group to which the metal-cutting machine belongs, the second is the type of device, its type, the third (and in some cases the fourth) is the main standard size of the unit.
Decoding the markings of metal-cutting machines
After the numbers listed in the model marking, there may be letters that determine whether the model of the metal-cutting machine has special characteristics. These characteristics of the device may include its level of accuracy or indication of modification. Often in the designation of a machine, the letter can be found after the first digit: this indicates that this is a modernized model, in the standard design of which some changes have been made.
As an example, you can decipher the markings of the 6M13P machine. The numbers in this designation indicate that we have a milling machine (“6”) of the first type (“1”), which belongs to the 3rd standard size (“3”) and allows processing with increased accuracy (letter “P” ). The letter “M” present in the marking of this device indicates that it has been modernized.
Automation levels
Types of lathes, as well as devices for any other purpose that are used in mass and large-scale production, are called aggregate. They received this name due to the fact that they are assembled from the same type of units (units): beds, working heads, tables, spindle units and other mechanisms. Completely different principles are used when creating machines that are necessary for small-scale and single-piece production. The design of such devices, which are highly versatile, can be completely unique.
CNC lathe
Classification of lathes (as well as equipment of any other categories) according to the level of automation implies their division into the following types:
- manual models, all operations on which are carried out manually;
- semi-automatic, in which part of the technological operations (installation of the workpiece, starting the device, removing the finished part) is performed manually (all other auxiliary operations are carried out in automatic mode);
- automatic, for which you only need to set processing parameters; they perform all other operations independently, in accordance with a given program;
- metal-cutting units with CNC (all processes on such machines are controlled by a special program that contains a coded system of numerical values);
- metal-cutting equipment belonging to the category of flexible automated modules.
The most prominent representatives of metal-cutting machines are CNC devices, the operation of which is controlled by a special computer program. Such a program, which is entered into the machine’s memory by its operator, determines almost all parameters of the unit’s operation: spindle speed, processing speed, etc.
Even the most compact desktop machines can be equipped with a CNC system
All types of metalworking machines equipped with a CNC system contain the following standard elements in their design.
- The operator's console (or console), through which a computer program that controls its operation is stored in the machine's memory. In addition, using such a remote control, you can manually control all parameters of the unit’s operation.
- The controller is an important element of the CNC system, with the help of which not only control commands are generated, transmitted to the working elements of the equipment, and the correctness of their execution is monitored, but also all the necessary calculations are made. Depending on the degree of complexity of the unit model, either a powerful compressor or a conventional microprocessor can be used as a controller to equip it.
- A screen or display that acts as a control and control panel for the operator. This element allows you to monitor the operation of a metal-cutting machine in real time, control the processing process, and, if necessary, quickly change parameters and settings.
The operating principle of metalworking machines equipped with a CNC system is simple. A program is first written that takes into account all the requirements for processing a specific workpiece, then the operator enters it into the machine controller using a special programmer. The commands embedded in such a program are sent to the working elements of the equipment, and after they are executed, the machine automatically turns off.
The use of metal-cutting machines equipped with numerical control allows processing with high accuracy and productivity, which is the reason for their active use to equip industrial enterprises that produce products in large series. Due to their high level of automation, such units are perfectly integrated into large automated lines.
The device of a screw-cutting lathe
Machine design
All machines belonging to the metalworking category have many common features in their design. In fact, the design and technical characteristics of such units must ensure the correct execution of two types of technological movements:
- the feed movement performed by the cutting fixture or the workpiece itself;
- the movement by which cutting is accomplished.
To perform these movements, as well as to ensure the stable functioning of all other elements of metalworking equipment, its design includes the following working parts:
- a control system responsible for starting and stopping the machine, monitoring all parameters of its operation;
- a unit with the help of which the movement from the electric motor is converted and transmitted to the actuator;
- directly the drive itself, which can be electrical, mechanical, pneumatic or hydraulic.
An important design element is also the units of metal-cutting equipment on which the cutting tool is installed and secured.
It is with the help of such units that the main function of the device is realized - processing parts made of metal. met-all.org
What accuracy classes are there and how are they designated?
As we have already found out, the error interval is determined by the accuracy class. This value is calculated and established by GOST and technical conditions. Depending on the specified error, it can be: absolute, reduced, relative, see table below
According to GOST 8.401-80 in the SI system, accuracy classes are usually marked with a Latin letter, often with the addition of an index marked with a number. The smaller the error, respectively, the smaller the number and the higher the letter value in the alphabet, the higher the accuracy.
Devices capable of performing many different measurements can be of more than two classes at the same time.
The accuracy class is indicated on the device body as a number circled, indicating the range of measurement errors as a percentage. For example, the number ② indicates a relative error of ±2% . If there is a check mark next to the symbol, this means that the scale length is used as an auxiliary determination of the error.
- 0,1, 0,2 – considered the highest class
- 0,5, 1 – more often used for devices in the mid-price category, for example, household ones
- 1,5, 2,5 – used for measuring instruments with low accuracy or indicators, analog sensors
Note. On the body of high-precision meters, the class may not be applied. The designation of such devices is usually done with special symbols.
State standards for laboratory balances
It should be noted that for laboratory scales, standards apply for scales intended for static (not dynamic) mass measurement in laboratories and enterprises. These standards do not apply to special-purpose scales, pharmaceutical scales, mass comparators, or scales that measure mass indirectly (not indirectly).
So, one of the first standards establishing the requirements for laboratory scales is the outdated GOST 24104-88 “General-purpose and standard laboratory scales” from 1988.
Further, already in the Russian Federation, in 2001, a new GOST 24104-2001 “Laboratory Balances” was adopted, in which the standards for laboratory scales were significantly changed, and the division of equipment into general-purpose scales and the so-called. the exemplary ones were removed in principle. In this regard, the accuracy classes of scales have undergone significant changes:
| Accuracy class according to GOST 24104-2001 | Accuracy class according to GOST 24104-88 |
| I "Special" | 1st grade, 2nd grade, 3rd grade (almost all) |
| II "High" | 4th grade |
| III "Medium" | Many devices are from GOST 29329-92 “Scales for static weighing”. |
The following changes have also been made:
- Introduction of new characteristics (division value (discreteness) “d”, smallest weighing limit (NmPV, Min), verification division price “e”, number of verification divisions “n”.
- Tests (0.5-hour and 4-hour) are established as a mandatory characteristic.
- The ability to operate multi-range scales (devices with several LELs) has been implemented. Structurally, each range is considered as a separate scale model with separate technical characteristics.
This GOST expired in 2010, and then it was replaced by the international standard for scales GOST OIML R 1 2011. It does not contain significant technical innovations and was created to comply with world (international) standards. This was an important milestone for manufacturers supplying weighing equipment for export.
Determination of error
Owners of measuring instruments are interested, first of all, in the maximum error characteristic of a pressure gauge. It depends not only on the accuracy class, but also on the measurement range. Thus, to obtain the error value, you need to make some calculations. For example, for a pressure gauge with a measurement range of 6 MPa and an accuracy class of 1.5, the error will be calculated using the formula 6*1.5/100=0.09 MPa.
It should be noted that only the main error can be calculated in this way.
Its value is determined by ideal operating conditions. It is influenced only by the design characteristics, as well as the assembly features of the device, for example, the accuracy of the graduation of divisions on the scale, the friction force in the measuring mechanism. However, this value may differ from the actual value, since there is also an additional error determined by the conditions in which the pressure gauge is operated. It can be affected by vibration of the pipeline or equipment, temperature, humidity level and other parameters.
Also, the accuracy of pressure measurement depends on another characteristic of the pressure gauge - the magnitude of its variation, which is determined during verification. This is the maximum difference in meter readings identified from the results of several measurements.
The magnitude of the variation largely depends on the design of the pressure gauge, namely on the method of balancing, which can be liquid (pressure from a liquid column) or mechanical (spring). Mechanical pressure gauges have a more pronounced variation, which is often due to additional friction due to poor lubrication or wear of parts, loss of spring elasticity and other factors.
Source:
3.4. Calculation of measurement system error
The measuring system is designed to perceive, process and store measurement information in the general case of heterogeneous physical quantities through various measuring channels (IC). Therefore, calculating the error of a measuring system comes down to estimating the errors of its individual IRs.
The resulting relative error of the IR will be
,
where x -
current value of the measured value;
—
the limit of a given channel measurement range at which the relative error is minimal; - relative errors calculated at the beginning and end of the range, respectively.
Since IR is a chain of various perceiving, converting and recording links, then to determine , ( x
) it is necessary, first of all, to estimate the standard deviation of the errors of these
m
links. Then the resulting standard deviation of the IR error will be
,
where are additional errors from n
influencing factors; ;— the limits of the permissible main error;— the quantile coefficient determined by the distribution law and the confidence probability of finding the error in a given interval.
Example 3.2.
Determine the error of the power measurement channel, the block diagram of which is shown in Fig.
3.10. Here CT and VT are current and voltage transformers, respectively; — power and current converters, respectively; K - switch; ADC is an analog-to-digital converter. Initial data: the relative error of the TT ,
given at the beginning of the measurement range, is , and at the end -; relative error of TN; The standard deviation error of power conversion consists of five components: the main error (1%); ripple errors (0.2%); additional error from changing cos φ (0.15%); errors from fluctuations in supply voltage (0.1%) and fluctuations in ambient temperature (0.6%); cos φ= 0.85; and from changes in ambient temperature; the error of a switch for 128 channels consists of three components: the error of the open key voltage drop (0.4%), the current leakage in each of the 127 channels closed by the key (0.13%) and the carrier frequency ripple (0.06%);
Rice. 3.10 Power measurement channel
Solution. 1. Considering that the law of error distribution is unknown, let us assume it to be uniform ( k
=1.73), and using formula (3.11) we find and.
For voltage transformer. Accepting the previous conditions,.