The main task of engineering design is the selection of the optimal profile section and structural material. It is necessary to find exactly the size that will ensure that the shape of the system is maintained with the minimum possible mass under the influence of load. For example, what kind of steel should be used as a span beam for a structure? The material may be used irrationally, installation will become more complicated and the structure will become heavier, and financial costs will increase. This question will be answered by such a concept as the elastic modulus of steel. It will also allow you to avoid these problems at a very early stage.
Table of material elasticity indicators
Before moving directly to this characteristic of steel, let us first consider, as an example and additional information, a table containing data on this value in relation to other materials. Data is measured in MPa.
Modulus of elasticity of various materials
As you can see from the table above, this value is different for different materials, and the indicators also differ, if we take into account one or another option for calculating this indicator. Everyone is free to choose exactly the option for studying indicators that suits them best. It may be preferable to consider Young's modulus, since it is most often used specifically to characterize a particular material in this regard.
After we have briefly reviewed the data on this characteristic of other materials, we will move directly to the characteristics of steel separately.
First, let's look at the hard numbers and derive different indicators of this characteristic for different types of steels and steel structures:
- Modulus of elasticity (E) for casting, hot-rolled reinforcement from steel grades called St.3 and St. 5 equals 2.1*106 kg/cm^2.
- For steels such as 25G2S and 30KhG2S this value is 2*106 kg/cm^2.
- For periodic wire and cold-drawn round wire, there is an elasticity value equal to 1.8 * 106 kg/cm^2. For cold-flattened reinforcement the indicators are similar.
- For strands and bundles of high-strength wire the value is 2·10 6 kg/cm^2
- For steel spiral ropes and ropes with a metal core, the value is 1.5·10 4 kg/cm^2, while for cables with an organic core this value does not exceed 1.3·10 6 kg/cm^2.
- The shear modulus (G) for rolled steel is 8.4·10 6 kg/cm^2.
- And finally, Poisson’s ratio for steel is equal to 0.3
These are general data given for types of steel and steel products. Each value was calculated in accordance with all physical rules and taking into account all existing relationships that are used to derive the values of this characteristic.
Below will be given all the general information about this characteristic of steel. Values will be given both by Young's modulus and by shear modulus, both in one unit of measurement (MPa) and in another (kg/cm2, newton*m2).
Steel and several different grades
| Material | Elastic modulus indicators (E, G; N*m2, kg/cm^2, MPa) |
| Steel | 20.6*10^10 newton*meter^2 |
| Carbon steel | E=(2.0…2.1)*10^5 MPa; G=(8.0…8.1)*10^4 MPa |
| Steel 45 | E=2.0*10^5 MPa; G=0.8*10^5 MPa |
| Steel 3 | E=2.1*10^5 MPa; G=0.8*10^5 MPa |
| Alloy steel | E=(2.1…2.2)*10^5 MPa; G=(8.0…8.1)*10^4 MPa |
The elasticity values of steel vary, since there are several modules at once, which are calculated and calculated in different ways. You can notice the fact that, in principle, the indicators do not differ greatly, which indicates in favor of different studies of the elasticity of various materials. But it’s not worth going too deep into all the calculations, formulas and values, since it’s enough to choose a certain elasticity value in order to focus on it in the future.
By the way, if you do not express all the values in numerical ratios, but take it immediately and calculate it completely, then this characteristic of steel will be equal to: E = 200,000 MPa or E = 2,039,000 kg/cm^2.
This information will help you understand the very concept of modulus of elasticity, as well as become familiar with the main values of this characteristic for steel, steel products, and also for several other materials.
It should be remembered that the elastic modulus indicators are different for different steel alloys and for different steel structures that contain other compounds. But even in such conditions, you can notice the fact that the indicators do not differ much. The elastic modulus of steel practically depends on the structure. and also on carbon content. The method of hot or cold processing of steel also cannot greatly affect this indicator.
Copper connections
Copper (I) oxide Cu2O3 and cuprous oxide (I) Cu2O , like other copper (I) compounds, are less stable than copper (II) compounds. Copper (I) oxide, or cuprous oxide Cu2O, occurs naturally as the mineral cuprite. In addition, it can be obtained as a precipitate of red copper(I) oxide by heating a solution of a copper(II) salt and an alkali in the presence of a strong reducing agent.
Copper(II) oxide, or copper oxide, CuO is a black substance found in nature (for example, as the mineral tenerite). It is obtained by calcination of copper (II) hydroxycarbonate (CuOH)2CO3 or copper (II) nitrate Cu(NO2)2. Copper(II) oxide is a good oxidizing agent.
Copper (II) hydroxide Cu(OH)2 precipitates from solutions of copper (II) salts under the action of alkalis in the form of a blue gelatinous mass. Even with low heating, even under water, it decomposes, turning into black copper (II) oxide. Copper(II) hydroxide is a very weak base. Therefore, solutions of copper (II) salts in most cases have an acidic reaction, and with weak acids copper forms basic salts.
Copper (II) sulfate CuSO4 in the anhydrous state is a white powder that turns blue when it absorbs water. Therefore, it is used to detect traces of moisture in organic liquids. An aqueous solution of copper sulfate has a characteristic blue-blue color. This color is characteristic of hydrated [Cu(H2O)4]2+ ions, therefore all dilute solutions of copper (II) salts have the same color, unless they contain any colored anions. From aqueous solutions, copper sulfate crystallizes with five molecules of water, forming transparent blue crystals of copper sulfate. Copper sulfate is used for electrolytic coating of metals with copper, for the preparation of mineral paints, and also as a starting material in the preparation of other copper compounds. In agriculture, a diluted solution of copper sulfate is used to spray plants and treat grain before sowing to destroy spores of harmful fungi.
Copper (II) chloride CuCl2. 2H2O. Forms dark green crystals, easily soluble in water. Very concentrated solutions of copper (II) chloride are green, diluted solutions are blue-blue.
Copper (II) nitrate Cu(NO3)2.3H2O. It is obtained by dissolving copper in nitric acid. When heated, blue copper nitrate crystals first lose water and then easily decompose, releasing oxygen and brown nitrogen dioxide, turning into copper (II) oxide.
Copper (II) hydroxycarbonate (CuOH)2CO3. It occurs naturally in the form of the mineral malachite, which has a beautiful emerald green color. It is artificially prepared by the action of Na2CO3 on solutions of copper (II) salts. 2CuSO4 + 2Na2CO3 + H2O = (CuOH)2CO3v + 2Na2SO4 + CO2^ Used to obtain copper (II) chloride, for the preparation of blue and green mineral paints, as well as in pyrotechnics.
Copper (II) acetate Cu (CH3COO)2.H2O. It is obtained by treating copper metal or copper(II) oxide with acetic acid. Usually it is a mixture of basic salts of various compositions and colors (green and blue-green). Under the name verdigris, it is used to prepare oil paint.
Complex copper compounds are formed as a result of the combination of doubly charged copper ions with ammonia molecules. A variety of mineral paints are obtained from copper salts. All copper salts are poisonous. Therefore, to avoid the formation of copper salts, copper utensils are coated on the inside with a layer of tin (tinned).
Elastic deformations. Young's modulus and Poisson's ratio. Energy of elastic deformation.
Determination of the initial modulus of elasticity of concrete B20, B25
All bodies are deformable
.
Changes caused by the actions of applied forces, during which bodies change shape and volume - deformations
.
Elastic
deformations
are deformations that disappear after the applied force ceases.
Plastic deformations
(
residual deformations
) – deformations that remain in the body (partially or completely) after the cessation of the applied force.
If the voltage
(force per unit area) does not exceed a certain value (
elastic limit
), then the deformation will be elastic.
Perfectly elastic
bodies – bodies that can only undergo elastic deformations.
For such bodies there is an unambiguous relationship between the forces and the deformations they cause
.
Small deformations
– deformations that obey
Hooke’s law
, according to which
deformations are proportional to the forces causing them isotropic anisotropic
Let there be two rods. We squeeze one, and squeeze the other with force (as in the picture). Let us draw a section perpendicular to the axis of the rod. To balance the rod, a force must act on its lower base. The lower and upper parts of the rod act on each other with equal force, because they are deformed. Ratio of force to cross-sectional area - stress
.
Tension
– tensile stress, .
Pressure
– compressive stress, where is the cross-sectional area. Pressure is negative voltage and vice versa.
– length of the undeformed rod. – length increment after application of force. That means full length. – relative elongation of the rod (if – relative compression).
For small elastic deformations, tension (pressure) is proportional to relative elongation (relative compression) -
(),
where is Young’s modulus
(constant, depending only on the material of the rod and its physical state).
Young's modulus
- the tension that must be applied to the rod so that its length doubles.
And the two formulas above are Hooke’s law
.
Let's calculate the elastic energy
stretched rod. Let us apply a tensile force to the rod and gradually (continuously and slowly) increase it from to . The elongation will vary from to . According to Hooke's law,
where is the elasticity coefficient
.
All work on stretching the rod will go towards increasing its elastic energy. Because in the final state, then , then for energy we get .
Under the action of a tensile or compressive force, not only the longitudinal but also the transverse dimensions of the rod change. If the force is tensile, then the transverse dimensions of the rod decrease. If it is compressive, then they increase.
– Poisson's ratio
.
It depends only on the material of the body in question. Young's modulus and Poisson's ratio fully characterize the elastic properties of an isotropic material. All other elastic deformations can be expressed through these coefficients.
Post Views: 4,220
Methods for determining and monitoring strength indicators of metals
The development of metallurgy and other related areas for the production of metal objects is due to the creation of weapons. At first they learned to smelt non-ferrous metals, but the strength of the products was relatively low. Only with the advent of iron and its alloys did the study of their properties begin.
The first swords were made quite heavy to give them hardness and strength. Warriors had to take them in both hands to control them.
Over time, new alloys appeared and production technologies were developed. Light sabers and swords came to replace heavy weapons. At the same time, tools were created.
With the increase in strength characteristics, tools and production methods were improved.
Types of loads
When using metals, different static and dynamic loads are applied. In the theory of strength, it is customary to define the following types of loads.
Compression - an acting force compresses an object, causing a decrease in length along the direction of application of the load. This deformation is felt by frames, supporting surfaces, racks and a number of other structures that can withstand a certain weight. Bridges and crossings, car and tractor frames, foundations and reinforcement - all these structural elements are under constant compression.
Tension - the load tends to lengthen the body in a certain direction. Lifting and transport machines and mechanisms experience similar loads when lifting and carrying loads.
Shear and shear - such loading is observed in the case of forces directed along the same axis towards each other. Connecting elements (bolts, screws, rivets and other hardware) experience this type of load. The design of housings, metal frames, gearboxes and other components of mechanisms and machines necessarily contains connecting parts. The performance of devices depends on their strength.
Torsion - if a pair of forces acting on an object are located at a certain distance from each other, then a torque occurs. These forces tend to produce torsional deformation. Similar loads are observed in gearboxes; the shafts experience just such a load. It is most often inconsistent in meaning. Over time, the magnitude of the acting forces changes.
Bending – a load that changes the curvature of objects is considered bending. Bridges, crossbars, consoles, lifting and transport mechanisms and other parts experience similar loading.
In the middle of the 17th century, materials research began simultaneously in several countries. A variety of methods have been proposed for determining strength characteristics. The English researcher Robert Hooke (1660) formulated the main provisions of the law on the elongation of elastic bodies as a result of the application of a load (Hooke's law). The following concepts were also introduced:
- Stress σ, which in mechanics is measured in the form of a load applied to a certain area (kgf/cm², N/m², Pa).
- Elastic modulus E, which determines the ability of a solid body to deform under loading (applying force in a given direction). Units of measurement are also defined in kgf/cm² (N/m², Pa).
The formula according to Hooke's law is written as ε = σz/E, where:
- ε – relative elongation;
- σz – normal stress.
Demonstration of Hooke's law for elastic bodies:
From the above dependence, the value of E for a certain material is derived experimentally, E = σz/ε.
The modulus of elasticity is a constant value that characterizes the resistance of a body and its structural material under normal tensile or compressive load.
In the theory of strength, the concept of Young's modulus of elasticity is adopted. This English researcher gave a more specific description of the methods of changing strength indicators under normal loads.
The elastic modulus values for some materials are given in Table 1.
Table 1: Modulus of elasticity for metals and alloys
| Name of material | Elastic modulus value, 10¹² Pa |
| Aluminum | 65…72 |
| Duralumin | 69…76 |
| Iron, carbon content less than 0.08% | 165…186 |
| Brass | 88…99 |
| Copper (Cu, 99%) | 107…110 |
| Nickel | 200…210 |
| Tin | 32…38 |
| Lead | 14…19 |
| Silver | 78…84 |
| Gray cast iron | 110…130 |
| Steel | 190…210 |
| Glass | 65…72 |
| Titanium | 112…120 |
| Chromium | 300…310 |
Modulus of elasticity of various materials
Gsm module for boiler
Elastic moduli for different materials have completely different values, which depend on:
- the nature of the substances that form the composition of the material;
- mono- or multicomponent composition (pure substance, alloy, etc.);
- structures (metallic or other type of crystal lattice, molecular structure, etc.);
- density of the material (distribution of particles in its volume);
- the processing to which it was subjected (firing, etching, pressing, etc.).
Its significance for bronze materials depends not only on the processing, but also on the chemical composition:
- bronze – 10.4 GPa;
- aluminum bronze during casting – 10.3 GPa;
- rolled phosphor bronze – 11.3 GPa.
Young's modulus of brass is much lower - 78.5-98.1. Rolled brass has the maximum value.
Copper itself in its pure form is characterized by a resistance to external influences that is much greater than its alloys - 128.7 GPa. Processing it also reduces the indicator, including rolling:
- cast – 82 GPa;
- rolled – 108 GPa;
- deformed – 112 GPa;
- cold drawn – 127 GPa.
Titanium (108 GPa), which is considered one of the strongest metals, has a value close to copper. But heavy but brittle lead shows only 15.7-16.2 GPa, which is comparable to the strength of wood.
For iron, the stress-to-strain ratio also depends on the method of its processing: cast - 100-130 or forged - 196.2-215.8 GPa.
Cast iron is known for its brittleness and has a stress-to-strain ratio of 73.6 to 150 GPa, which is consistent with its type. Whereas for steel, the elastic modulus can reach 235 GPa.
Elastic moduli of some materials
The values of strength parameters are also influenced by the shape of the products. For example, for a steel rope, calculations are carried out, which take into account:
- its diameter;
- lay pitch;
- lay angle.
Interestingly, this figure for a rope will be significantly lower than for a wire of the same diameter.
It is worth noting the strength and non-metallic materials. For example, among the Young's moduli of wood, pine has the lowest - 8.8 GPa, but the group of hardwoods, which are united under the name "iron wood", has the highest - 32.5 GPa, oak and beech have equal indicators - 16.3 GPa.
Among building materials, the resistance to external forces of seemingly durable granite is only 35-50 GPa, while even glass is 78 GPa. Concrete is inferior to glass - up to 40 GPa, limestone and marble, with values of 35 and 50 GPa, respectively.
Flexible materials such as rubber can withstand axial loads ranging from 0.0015 to 0.0079 GPa.
Allowable tensile stress in some materials
From life experience we know that different materials resist changes in shape in different ways. The strength characteristics of crystalline and other solids are determined by the forces of interatomic interaction. As interatomic distances increase, the forces that attract atoms to each other also increase. These forces reach a maximum at a certain amount of stress, equal to approximately one tenth of Young's modulus.
Tensile test
This value is called theoretical strength; when it is exceeded, the destruction of the material begins. In reality, destruction begins at lower values, since the structure of real samples is heterogeneous. This causes an uneven distribution of stresses, and destruction begins from those areas where the stresses are maximum.
| Materials | σrast | |
| Bor | 5700 | 0,083 |
| Graphite | 2390 | 0,023 |
| Sapphire | 1495 | 0,030 |
| Steel wire | 415 | 0,01 |
| Fiberglass | 350 | 0,034 |
| Structural steel | 60 | 0,003 |
| Nylon | 48 | 0,0025 |
These numbers are taken into account by designers when choosing the material for parts of a future product. Strength calculations are also carried out using them. For example, cables used for lifting and transport operations must have a tenfold safety margin. They are periodically checked by hanging a load ten times greater than the cable's rated load capacity.
The safety margins built into critical structures are also multiple.
Factors affecting Young's modulus
Prices for stainless steel fencing per meter
Young's modulus is the main characteristic of concrete that determines its strength. Due to its size, designers carry out calculations of the material’s resistance to various types of loads. The indicator is influenced by many factors:
- quality and quantity of aggregates;
- concrete class;
- air humidity and temperature;
- time of exposure to load factors;
- reinforcement.
PHOTO: dostroy.comThe modulus of elasticity allows designers to correctly calculate the load
Quality and quantity of aggregates
The quality of concrete depends on its aggregates. If the components have low density, accordingly, Young's modulus will be small. The elasticity of the material increases several times if heavy fillers are used.
PHOTO: russkaya-banja.ruLarge components increase elasticity characteristics
PHOTO: ivdon.ru Graph of the dependence of the tensile strength of a material on cement stone
Material class
The class of concrete also influences the coefficient: the lower it is, the lower the value of the elastic modulus. For example:
- the elastic modulus of B10 corresponds to a value of 19;
- B15 – 24;
- V-20 – 27.5;
- B25 – 30;
- the indicator for B30 increases to a value of 32.5.
PHOTO: buildingclub.ruDependence on concrete class
How do humidity and temperature values affect the indicator?
The increase in deformations and decrease in the elastic properties of the material are influenced by:
- increase in air temperature;
- increase in solar activity.
Under the influence of negative environmental factors, the internal energy of the material increases, this leads to linear expansion of concrete and, accordingly, to an increase in plasticity.
The creep of the material is affected by moisture, leading to a change in elastic characteristics. The higher the water vapor content, the lower the coefficient.
PHOTO: betonpro100.ru The influence of humidity on the creep of concrete
Load exposure time and mixture hardening conditions
The elasticity index is affected by the time of exposure to the load:
- with an instantaneous force on a concrete structure, the deformability is directly proportional to the magnitude of the external load;
- with prolonged exposure, the coefficient values decrease.
During the research, it was noted that if concrete hardens naturally, its elastic modulus is higher, in contrast to steaming the material under various conditions. This is explained by the fact that when external conditions are used, large quantities of voids and pores are formed in concrete, worsening its elastic properties.
PHOTO: udarnik.spb.ru Dependence of elastic moduli on various factors
Age of concrete and reinforcement of the structure
The strength of concrete is directly dependent on its age; over time, the indicator only increases. Another factor that has a positive effect on the elastic modulus of concrete is reinforcement, which prevents deformation of the material.
PHOTO: 63-ds.netsamara.ru For structures that will be operated under heavy loads, it is necessary to install a metal grid
Modulus of elasticity of wood
Wood is considered elastic if, after removing the force that bends it, it returns to its original shape. Elasticity has a limit. It is achieved when, when bent, a wooden part or product retains its final shape. Simply put, the elastic limit of the board is reached at the moment when it breaks. The properties of elasticity and flexibility are not identical. Flexibility is the ability to change shape under the influence of external influences. Elasticity – the ability to regain lost shape. Wood with a high modulus is necessary for making sports equipment and furniture. The most elastic wood is from species such as ash, beech, hazel, and larch.
To describe the ability to return to its original shape, the following physical quantities are used:
- modulus of elasticity E;
- deformation coefficient µ;
- shear modulus G.
In general, we can say that when a force is applied along wood fibers, the modulus of elasticity is 20-25 times higher than if the same force acts across the fibers. If the force acts perpendicular to the direction of the fibers and is directed radially, then this figure is 20-50% greater than when the same force is applied in the tangential direction.
Below we will consider in more detail these physical quantities that determine the ability of wood to return to its original shape when the deforming force is removed.
Modulus of elasticity of basic wood species
The modulus of elasticity in physics is considered as a single name for a complex of physical quantities that characterize the ability of a solid body (in our case, wood) to elastically deform if some force is applied to it.
The modulus of elasticity of wood (E) is the relationship between normal stresses and relative deformations. It is measured in MPa or kgf/cm2 (1 MPa=10.197 kgf/cm2) There are several types:
- along the fibers of Ea.
- across the grain (tangential) Et.
- across the fibers (radial) Er.
- modulus of elasticity in bending Ebg.
Table. Information on the most commonly used breeds.*
Transverse deformation coefficients of main wood species
When a load is applied, in addition to longitudinal deformation along the fibers, transverse deformation also appears during bending.
The coefficients of this type of deformation are given in the table:
Shear modulus of basic wood species
Shear modulus is the proportionality coefficient between shear stresses and angular deformations of wood.
Shear modulus data for the main rocks is given below:
Plasticity of wood
A tree is capable of changing its shape under pressure without destruction, and retaining it after the pressure is removed. This property is called plasticity. Plasticity depends on the same criteria as elasticity, only in the opposite direction. For example, the higher the moisture content of wood, the more plastic it is, but less elastic.
The plasticity of wood is increased with the help of special processing. By steaming or boiling it in water, we obtain a more plastic material, which is then used to make furniture and sleigh runners. The highest plasticity is found in beech, elm, ash, and oak. This property is due to the structure of the conductive system of these rocks. Beech, for example, has many large medullary rays that bend the wood fibers. The vessels located in groups in the annual layers of elm, oak, and ash are strongly compressed by denser late wood, so the plasticity of these species is high.
Unit of measurement and formulas
The SI unit of Young's modulus is Newton per meter squared (N/m²), i.e. Pascal (Pa).
Formulas
There are several formulas from which you can calculate Young's modulus. For example, Hooke's law.
Hooke's law
You can calculate Young's modulus using these formulas (we will do this using an example). Because of this law, there are several interesting equalities that can be useful for calculations.
Hooke's law (this one describes phenomena in the body, in differential form):
- σ - mechanical stress
- E - Young's modulus (elastic modulus)
- ε—relative elongation
Hooke's Law (this one describes phenomena in the body)
- Fupr - elastic force
- k × Δl—body elongation
- Fupr - elastic force
- E - Young's modulus (elastic modulus)
- S - cross-sectional area
- l - initial body length
- Δl - body elongation
- Fpr/S - mechanical stress, denoted as σ
- Δl/l - relative elongation, denoted as ε
It should be noted that this law applies to the point where the material is irreversibly deformed and no longer returns to its original shape. At what point this happens depends on the material. If the material is very stiff (meaning a high elastic modulus reading), then this point may coincide with rupture/deformation.
Other formulas for calculating Young's modulus (elastic modulus)
- E - Young's modulus (elastic modulus)
- k - body stiffness
- l is the original length of the rod
- S - cross-sectional area
Or you can express k (body stiffness):
- k - body stiffness
- E - Young's modulus (elastic modulus)
- S - cross-sectional area
- l is the initial length of the rod/body
An example of solving the problem (via Hooke's law):
A wire 2.5 meters long and with a cross-sectional area of 2.5 millimeters² is elongated by 1 millimeter under a force of 50 newtons. Determine Young's modulus.
- l = 2.5 m
- F=50H
- E = ?
We will search through Hooke's law (σ = E × ε).
Remember from Hooke's law:
σ = F / S (remember that Fpr/S is mechanical stress, denoted as σ)
ε = Δl/l (and this is the relative elongation, denoted as ε)
Substitute into the formula (σ = E × ε):
For example, in our table, cadmium has such a Young’s modulus.
Young's modulus of elasticity and shear, Poisson's ratio values (Table)
Elastic properties of bodies
Below are reference tables for commonly used constants; if two of them are known, then this is quite sufficient to determine the elastic properties of a homogeneous isotropic solid.
Young's modulus or modulus of longitudinal elasticity in dyn/cm2.
Shear modulus or torsional modulus G in dyn/cm2.
Compressive modulus or bulk modulus K in dynes/cm2.
Compressibility volume k=1/K/.
Poisson's ratio µ is equal to the ratio of transverse relative compression to longitudinal relative tension.
For a homogeneous isotropic solid material, the following relationships between these constants hold:
G = E / 2(1 + μ) - (α)
μ = (E / 2G) - 1 - (b)
K = E / 3(1 - 2μ) - (c)
Poisson's ratio has a positive sign and its value is usually between 0.25 and 0.5, but in some cases it can go beyond these limits. The degree of agreement between the observed values of µ and those calculated using formula (b) is an indicator of the isotropy of the material.
Tables of Young's Modulus of Elasticity, Shear Modulus and Poisson's Ratio
Values calculated from relations (a), (b), (c) are given in italics.
| Material at 18°C | Young's modulus E, 1011 dynes/cm2. | Shear modulus G, 1011 dynes/cm2. | Poisson's ratio µ | Modulus of bulk elasticity K, 1011 dynes/cm2. |
| Aluminum | 7,05 | 2,62 | 0,345 | 7,58 |
| Bismuth | 3,19 | 1,20 | 0,330 | 3,13 |
| Iron | 21,2 | 8,2 | 0,29 | 16,9 |
| Gold | 7,8 | 2,7 | 0,44 | 21,7 |
| Cadmium | 4,99 | 1,92 | 0,300 | 4,16 |
| Copper | 12,98 | 4,833 | 0,343 | 13,76 |
| Nickel | 20,4 | 7,9 | 0,280 | 16,1 |
| Platinum | 16,8 | 6,1 | 0,377 | 22,8 |
| Lead | 1,62 | 0,562 | 0,441 | 4,6 |
| Silver | 8,27 | 3,03 | 0,367 | 10,4 |
| Titanium | 11,6 | 4,38 | 0,32 | 10,7 |
| Zinc | 9,0 | 3,6 | 0,25 | 6,0 |
| Steel (1% C) 1) | 21,0 | 8,10 | 0,293 | 16,88 |
| (soft) | 21,0 | 8,12 | 0,291 | 16,78 |
| Constantan 2) | 16,3 | 6,11 | 0,327 | 15,7 |
| Manganin | 12,4 | 4,65 | 0,334 | 12,4 |
| 1) For steel containing about 1% C, elastic constants are known to change during heat treatment. 2) 60% Cu, 40% Ni. |
The experimental results given below are for common laboratory materials, mainly wires.
| Substance | Young's modulus E, 1011 dynes/cm2. | Shear modulus G, 1011 dynes/cm2. | Poisson's ratio µ | Modulus of bulk elasticity K, 1011 dynes/cm2. |
| Bronze (66% Cu) | -9,7-10,2 | 3,3-3,7 | 0,34-0,40 | 11,2 |
| Copper | 10,5-13,0 | 3,5-4,9 | 0,34 | 13,8 |
| Nickel silver1) | 11,6 | 4,3-4,7 | 0,37 | — |
| Glass | 5,1-7,1 | 3,1 | 0,17-0,32 | 3,75 |
| Glass yen crowns | 6,5-7,8 | 2,6-3,2 | 0,20-0,27 | 4,0-5,9 |
| Jena flint glass | 5,0-6,0 | 2,0-2,5 | 0,22-0,26 | 3,6-3,8 |
| Welding iron | 19-20 | 7,7-8,3 | 0,29 | 16,9 |
| Cast iron | 10-13 | 3,5-5,3 | 0,23-0,31 | 9,6 |
| Magnesium | 4,25 | 1,63 | 0,30 | — |
| Phosphor bronze2) | 12,0 | 4,36 | 0,38 | — |
| Platinoid3) | 13,6 | 3,6 | 0,37 | — |
| Quartz threads (floating) | 7,3 | 3,1 | 0,17 | 3,7 |
| Soft vulcanized rubber | 0,00015-0,0005 | 0,00005-0,00015 | 0,46-0,49 | — |
| Steel | 20-21 | 7,9-8,9 | 0,25-0,33 | 16,8 |
| Zinc | 8,7 | 3,8 | 0,21 | — |
| 1) 60% Cu, 15% Ni, 25% Zn 2) 92.5% Cu, 7% Sn, 0.5% P 3) Nickel silver with a small amount of tungsten. |
| Substance | Young's modulus E, 1011 dynes/cm2. | Substance | Young's modulus E, 1011 dynes/cm2. |
| Zinc (pure) | 9,0 | Oak | 1,3 |
| Iridium | 52,0 | Pine | 0,9 |
| Rhodium | 29,0 | Red tree | 0,88 |
| Tantalum | 18,6 | Zirconium | 7,4 |
| Invar | 17,6 | Titanium | 10,5-11,0 |
| Alloy 90% Pt, 10% Ir | 21,0 | Calcium | 2,0-2,5 |
| Duralumin | 7,1 | Lead | 0,7-1,6 |
| Silk threads1 | 0,65 | Teak | 1,66 |
| Web2 | 0,3 | Silver | 7,1-8,3 |
| Catgut | 0,32 | Plastics: | |
| Ice (-20C) | 0,28 | Thermoplastic | 0,14-0,28 |
| Quartz | 7,3 | Thermoset | 0,35-1,1 |
| Marble | 3,0-4,0 | Tungsten | 41,1 |
| 1) Reduces rapidly with increasing load 2) Detects noticeable elastic fatigue |
| Temperature coefficient (at 150C) Et=E11 (1-ɑ (t-15)), Gt=G11 (1-ɑ (t-15)) | Compressibility k, bar-1 (at 7-110C) | |||
| ɑ, for E | ɑ, for G | |||
| Aluminum | 4,8*10-4 | 5,2*10-4 | Aluminum | 1,36*10-6 |
| Brass | 3,7*10-4 | 4,6*10-4 | Copper | 0,73*10-6 |
| Gold | 4,8*10-4 | 3,3*10-4 | Gold | 0,61*10-6 |
| Iron | 2,3*10-4 | 2,8*10-4 | Lead | 2,1*10-6 |
| Steel | 2,4*10-4 | 2,6*10-4 | Magnesium | 2,8*10-6 |
| Platinum | 0,98*10-4 | 1,0*10-4 | Platinum | 0,36*10-6 |
| Silver | 7,5*10-4 | 4,5*10-4 | Flint glass | 3,0*10-6 |
| Tin | — | 5,9*10-4 | German glass | 2,57*10-6 |
| Copper | 3,0*10-4 | 3,1*10-4 | Steel | 0,59*10-6 |
| Nickel silver | — | 6,5*10-4 | ||
| Phosphor bronze | — | 3,0*10-4 | ||
| Quartz threads | -1,5*10-4 | -1,1*10-4 |
Hooke's Law in Mathematical Form
Hooke's formulation, which we cited above, makes it possible to write it in the following form:
,
where the change in the length of a body due to compression or stretching, F is the force applied to the body and causes deformation (elastic force), k is the elasticity coefficient, measured in N/m.
It should be remembered that Hooke's law is valid only for small stretches.
We also note that it has the same appearance when stretched and compressed. Considering that force is a vector quantity and has a direction, then in the case of compression, the following formula will be more accurate:
, but again, it all depends on where the axis relative to which you are measuring will be directed.
What is the fundamental difference between compression and extension? Nothing if it is insignificant.
The degree of applicability can be considered as follows:
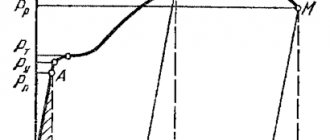
Let's pay attention to the graph. As we can see, with small stretches (the first quarter of the coordinates), for a long time the force with the coordinate has a linear relationship (red straight line), but then the real relationship (dotted line) becomes nonlinear, and the law ceases to be true
In practice, this is reflected by such strong stretching that the spring stops returning to its original position and loses its properties. With even greater stretching, a fracture occurs and the structure of the material is destroyed.
With small compressions (third quarter of the coordinates), for a long time the force with the coordinate also has a linear relationship (red line), but then the real relationship (dotted line) becomes nonlinear, and everything stops working again. In practice, this is reflected by such strong compression that heat begins to be released and the spring loses its properties. With even greater compression, the coils of the spring stick together and it begins to deform vertically, and then completely melt.
As you can see, the formula expressing the law allows you to find the force, knowing the change in the length of the body, or, knowing the elastic force, measure the change in length:
Also, in some cases, you can find the elasticity coefficient. To understand how this is done, consider an example task:
A dynamometer is connected to the spring. It was stretched using a force of 20 Newton, which is why it became 1 meter long. Then they released her, waited until the vibrations stopped, and she returned to her normal state. In normal condition, its length was 87.5 centimeters. Let's try to find out what material the spring is made of.
Given:
Solution:
Let's find the numerical value of the spring deformation:
Let's write down:
From here we can express the value of the coefficient:
Looking at the table, we can find that this indicator corresponds to spring steel.
Content
All bodies on Earth are affected by gravity. They all tend to fall down under its influence. But it's not like everything in the world is on the ground.
The food on your plate is affected by gravity, but it does not fall through the plate. The textbook on your desk is also affected by gravity. But this does not press the table to the floor.
This means that there is a certain force that balances the force of gravity. In this lesson we will learn what kind of power this is.
Effect of radioactive irradiation on changes in mechanical properties
Radiation exposure affects different materials differently. Irradiation of materials of inorganic origin in its effect on mechanical characteristics and plasticity characteristics is similar to a decrease in temperature: with an increase in the dose of radioactive irradiation, the tensile strength and especially the yield strength increase, and the plasticity characteristics decrease.
Irradiation of plastics also leads to an increase in fragility, and irradiation has different effects on the tensile strength of these materials: on some plastics it has almost no effect (polyethylene), in others it causes a significant decrease in tensile strength (katamen), and in others it increases the tensile strength (selectron ).
Parameters on which the elasticity of wood depends
The modulus of elasticity of wood is a variable parameter, its value is influenced by:
- Humidity. The elasticity of wood is inversely related to humidity. That is, with high wood humidity, its ability to return to its original shape will be minimal.
- Straightness. If the fibers are arranged in a tortuous, random manner, then its ability to restore shape will be noticeably lower than that of a straight layer.
- Density. Low density wood is not as resilient as denser wood.
- Age of the tree. The wood of an old tree is more elastic than a young one.
- Natural features of wood. Coniferous trees have single-row small core rays, so their wood is more elastic, although the specific gravity of such species is not high.
- The age of the wood itself. The younger layers of the tree trunk are called sapwood, those that are located closer to the center, and, accordingly, older ones are called the core. The sapwood is more resilient than the heartwood.
Characteristics of copper
- Copper density - 8.93*103kg/m3;
- Specific gravity of copper - 8.93 g/cm3;
- The specific heat capacity of copper at 20oC is 0.094 cal/deg;
- The melting point of copper is 1083oC;
- Specific heat of fusion of copper - 42 cal/g;
- The boiling point of copper is 2600oC;
- Linear expansion coefficient of copper
- (at a temperature of about 20oC) - 16.7 * 106 (1/deg);
- Thermal conductivity coefficient of copper is 335 kcal/m*hour*deg;
- Copper resistivity at 20oC - 0.0167 Ohm*mm2/m;
Mechanical properties
Only when working in tension or compression does the (Young's) modulus of elasticity help to predict the behavior of a particular material. But for bending, shearing, crushing and other loads, you will need to enter additional parameters:
Read also: A device that looks for wires in the wall
- Stiffness is the product of the profile cross-section and the elastic modulus. By this value one can judge the plasticity of the structural unit as a whole, and not about the material separately. The unit of measurement is kilograms force.
- Longitudinal elongation is the ratio of the absolute elongation of the sample material to its total length. For example, some force was applied to a rod whose length is 200 millimeters. As a result, it became shorter by 5 millimeters. As a result, the relative elongation will be equal to 0.05. This quantity is dimensionless. For more convenient perception, it is sometimes converted into percentages.
- Transverse relative elongation is calculated in the same way as longitudinal relative elongation, but instead of length, the diameter of the rod is taken. It was experimentally found that for a larger amount of material, the transverse elongation is approximately 4 times less than the longitudinal elongation.
- Poisson's ratio. This is the ratio of relative longitudinal to relative transverse strain. Using this value, it is possible to fully describe the changes in shape under the influence of load.
- The shear modulus describes the elastic properties under the influence of tangential properties on the sample. In other words, when the force vector is directed towards the surface of the body at 90 degrees. An example of such loads is the work of nails under crushing, rivets under shear, etc. This parameter is related to the viscosity of the material.
- The bulk modulus of elasticity characterizes the change in the volume of a sample for a versatile uniform application of load. This value is the ratio of volumetric pressure to volumetric compressive strain. As an example, we can consider a material lowered into water, which is subject to liquid pressure over its entire area.
In addition to all of the above, it is worth mentioning that some materials have different mechanical properties depending on the direction of the load. Such materials are called anisotropic. Examples of this are fabrics, some types of stone, laminated plastics, wood, etc.
Isotropic materials have the same mechanical properties and elastic deformation in any direction. Such materials include metals: aluminum, copper, cast iron, steel, etc., as well as rubber, concrete, natural stones, non-laminated plastics.
Basic information
Young's modulus (also called the longitudinal modulus of elasticity and the modulus of elasticity of the first kind) is an important mechanical characteristic of a substance. It is a measure of resistance to longitudinal deformation and determines the degree of rigidity. It is designated as E; measured in n/m 2 or in Pa.
This important coefficient is used when calculating the rigidity of workpieces, assemblies and structures, and in determining their resistance to longitudinal deformations. Substances used for the manufacture of industrial and building structures, as a rule, have very large E values. And therefore, in practice, E values for them are given in gigaPascals (10 12 Pa)
The value of E for rods can be calculated; for more complex structures it is measured during experiments.
Approximate values of E can be obtained from the graph constructed during tensile tests.
Tensile test schedule
E is the quotient of normal stress σ divided by relative elongation ε.
Hooke's law can also be formulated using Young's modulus.
Modulus of elasticity of plywood
Plywood is a building material made by gluing together several layers of wood veneer. She is very popular, and for good reason. In addition to aesthetic value, plywood has a number of parameters that distinguish it among materials for construction. After undergoing processing, plywood acquires strength, elasticity, and moisture resistance.
The characteristics of plywood are influenced by many factors:
- the type of wood used for veneer;
- initial state of raw materials;
- humidity of the plywood itself;
- the type and composition of the glue used to join the veneer layers;
- pre-treatment technology.
For plywood, the modulus of elasticity and all relevant coefficients are also calculated.
The important thing is that the modulus of elasticity of plywood and other indicators are higher than that of the wood from which it was made. The modulus of elasticity of wood must be calculated before constructing roofing and rafter systems.
Knowledge of the internal forces appearing in building materials is important for the safety and durability of the building. The ability to return a lost shape is significant when choosing the material for the handles of percussion instruments and weapon stocks
The modulus of elasticity of wood must be calculated before constructing roofing and rafter systems.
Knowledge of the internal forces appearing in building materials is important for the safety and durability of the building. The ability to return a lost shape is significant when choosing the material for the handles of percussion instruments and weapon stocks
Material tensile strength
This is the limit of the stress that occurs, after which the sample begins to fail.
Static tensile strength is measured under prolonged application of deforming force, dynamic - under short-term, impact nature of such force. For most substances the dynamic limit is greater than the static limit.
Read also: Makita hr2450 rotary hammer do-it-yourself repair
Tensile strength tool
In addition, there are limits to the material's compressive and tensile strength. They are determined experimentally on a test bench, by stretching or compressing samples with powerful hydraulic machines equipped with precise dynamometers and pressure meters. If it is impossible to achieve the required pressure hydraulically, a directed explosion in a sealed capsule is sometimes used.
General concept
The modulus of elasticity (also known as Young's modulus) is one of the indicators of the mechanical properties of a material, which characterizes its resistance to tensile deformation. In other words, its value shows the ductility of the material. The greater the elastic modulus, the less any rod will stretch, all other things being equal (load magnitude, cross-sectional area, etc.).
In the theory of elasticity, Young's modulus is denoted by the letter E. It is an integral part of Hooke's law (the law on the deformation of elastic bodies). Connects the stress arising in the material and its deformation.
According to the international standard system of units, it is measured in MPa. But in practice, engineers prefer to use the dimension kgf/cm2.
The elastic modulus is determined experimentally in scientific laboratories. The essence of this method is to tear dumbbell-shaped samples of material using special equipment. Having found out the stress and elongation at which the sample failed, divide these variables by each other, thereby obtaining Young's modulus.
Let us immediately note that this method is used to determine the elastic moduli of plastic materials: steel, copper, etc. Brittle materials - cast iron, concrete - are compressed until cracks appear.
Additional characteristics of mechanical properties
The modulus of elasticity makes it possible to predict the behavior of a material only when working in compression or tension. In the presence of such types of loads as crushing, shear, bending, etc., additional parameters will need to be introduced:
- Stiffness is the product of the elastic modulus and the cross-sectional area of the profile. By the value of rigidity, one can judge the plasticity not of the material, but of the structure as a whole. Measured in kilograms of force.
- Relative longitudinal elongation shows the ratio of the absolute elongation of the sample to the total length of the sample. For example, a certain force was applied to a rod 100 mm long. As a result, it decreased in size by 5 mm. Dividing its elongation (5 mm) by the original length (100 mm) we obtain a relative elongation of 0.05. A variable is a dimensionless quantity. In some cases, for ease of perception, it is converted to percentages.
- Relative transverse elongation is calculated similarly to the point above, but instead of length, the diameter of the rod is considered here. Experiments show that for most materials, transverse elongation is 3-4 times less than longitudinal elongation.
- The Punch ratio is the ratio of the relative longitudinal strain to the relative transverse strain. This parameter allows you to fully describe the change in shape under the influence of load.
- The shear modulus characterizes the elastic properties when the sample is exposed to tangential stresses, i.e., in the case when the force vector is directed at 90 degrees to the surface of the body. Examples of such loads are the work of rivets in shear, nails in crushing, etc. By and large, the shear modulus is associated with such a concept as the viscosity of the material.
- The bulk modulus of elasticity is characterized by a change in the volume of the material for uniform, versatile application of load. It is the ratio of volumetric pressure to volumetric compressive strain. An example of such work is a sample lowered into water, which is subject to liquid pressure over its entire area.
In addition to the above, it should be mentioned that some types of materials have different mechanical properties depending on the direction of loading. Such materials are characterized as anisotropic. Vivid examples are wood, laminated plastics, some types of stone, fabrics, etc.
Isotropic materials have the same mechanical properties and elastic deformation in any direction. These include metals (steel, cast iron, copper, aluminum, etc.), non-laminated plastics, natural stones, concrete, rubber.
SEARCH
E - reduced Young's modulus, taken equal to the modulus of elasticity of steel [p.71]
Deformation properties. Modulus R. (E) under slight tension is 4-5 decimal orders lower than Young’s modulus for steel [respectively 0.5- [p.158]
YOUNG'S MODULE OF CARBON AND ALLOY STEEL AT DIFFERENT TEMPERATURES [p.16]
It should be noted that the high elasticity of rubber is completely different from the elastic deformations of crystalline substances or metals, which constitute only a few percent of the original dimensions, while rubber can be stretched 10 times.
The stresses required for deformation also differ sharply.
The elastic modulus (or Young's modulus) E, which characterizes the relationship between the applied stress and the relative elongation of the sample, is about 20,000 kg/mm for steel, about 6,000 kg/mm for glass, and only about [p.228]
When designing, it is important to establish the distribution of structural deformations that arise during operation under the influence of applied stresses. Stresses can arise from pressure exerted by a liquid or gas, fluid flow, or non-uniform thermal expansion due to changes in temperature. Elastic properties are often considered to be independent of structure, but there are situations where this statement becomes incorrect. Individual grains of metal crystals are anisotropic with respect to elastic properties. Thus, the elastic constants depend on the orientation of the grain relative to the orientation of the applied stresses. During the manufacturing process of parts, a preferential orientation of individual grains may arise, which creates elastic anisotropy. It is very likely that different degrees of preferential orientation lead to a fairly wide scatter of data on the elastic properties of metals and alloys. Due to the fact that this scatter can cause errors, reaching 20% in some cases when calculating deformations, this topic is discussed in detail in this paragraph. Table 3, 4.5,8 is just an example of the type of information found in the literature. It can be assumed, for example, that steels with a 5-9% chromium content should have approximately the same values of Young's modulus as steels in which the chromium content is close to the specified one. [p.196]
The strength of steels changes significantly when moving to high temperatures. Thus, the tensile strength of chromium-nickel steel type 18-8 drops from 7000 to 4000 kgf/cm at 700 °C to 2000 kgf/cm at 800 °C. The Young's modulus of carbon and alloy steels decreases by 30% when heated from 20 to 500 °C. [p.19]
Sometimes a metal plate is placed between two piezoelectric elements to increase strength [318].
The natural frequency of the transducer can be increased by placing two passive (for example, steel) plates on either side of a bimorph transducer made of two piezo plates.
This is explained by the fact that the Young’s modulus of steel is much greater than that of piezoceramics, and the flexural rigidity of the structure is determined mainly by its [p.70]
The hardness of a substance can be estimated using Young's modulus, which is the ratio of the applied stress (or force per unit area) and the corresponding deformation or elongation.
Typical values of Young's modulus for various materials are presented in Fig. 7.1. At one end of the scale are inorganic crystalline materials, such as diamond, quartz, steel, etc., whose moduli [p.
131]
Even more impressive is the difference in the force required to effect the deformation.
To elongate a steel wire with a diameter of 1 mm by 1%, a load of 1600 N (twice the average weight of a person) is required, and to elongate a rubber thread of the same diameter by the same amount, a load of less than 10 N is required.
The so-called Young's modulus (stress to elongation ratio) for steel is 100,000 times greater than for rubber. [p.45]
Characteristics of steels and alloys at room temperature and oscillation frequency of 20 kHz (p - density E - Young's modulus Spr - speed of sound pc - wave impedance [p.115]
It should be noted that titanium of unreasonably large thickness is often used for communications, which is not caused by either strength or corrosion requirements. Often, ideas that have developed as a result of many years of work with steel are transferred to titanium as a structural material.
Thus, when replacing steel communications with titanium ones, titanium of the same thickness as steel is used. The large consumption of titanium specifically for communications is explained to some extent by this reason.
For example, wet chlorine collectors at factories are made from titanium sheets 3-5 mm thick (only at two enterprises these collectors are made from sheets 2 mm thick, but this is also a significant thickness). Abroad, titanium with a thickness of 0.8–1.0 mm is used for these purposes.
Due to the fact that the Young's modulus of titanium is insignificant, during calculations you should pay attention to the possible deflection of pipes, and during installation - to the fastening of pipelines. [p.156]
Interesting observations are published by Fiers-Vickers, claiming that stainless austenitic steels (chromium and chromium-nickel) show a drop in Young's modulus of approximately 1% for every 30° increase in temperature. In particularly severe operating conditions, it is best to contact steel suppliers for information. [p.670]
Vulcanized rubber is capable of experiencing reversible deformations of hundreds of percent with a very low elastic modulus. (Young's modulus for steel 20,000-22,000 kg/cm, for rubber [p.10]
It should be noted that the high elasticity of rubber is completely different from the elastic deformations of crystalline substances or metals, which amount to only a few percent of the original dimensions, while rubber can be stretched to tenfold elongations.
The stresses required for deformation also differ sharply.
The elastic modulus (or Young's modulus) E, which characterizes the relationship between the applied stress and the relative elongation of the sample, is about 20,000 kg/mm for steel, about 6000 kg/mm for glass, and only about 0.1 kg/mm for rubber. mm". These differences are explained by the fact that during elastic deformation of crystals, only small changes occur in the average distances between molecules and valence distances between atoms, associated with significant changes in internal energy; on the contrary, with pure highly elastic deformation, large elongations occur without changes in valence distances and the internal energy is constant. [p.272]
In practice, optical fibers with a two-layer coating have become increasingly used, in which the first layer is made of a soft (buffer) layer with a low (1–2 MPa) Young’s modulus, and the second layer with a high Young’s modulus from 0.1 to 4 GPa [52] [ p.101]
In formula (A1.2), the initial deformation 5 is usually (but not necessarily) taken to be the value of some conditionally elastic deformation, which determines the beginning of the plastic flow of the material.