Wire cross-section: concept, area, formula and diameter table

Any body through which electric current flows exhibits a certain resistance to it. The property of a conductor material to prevent electric current from passing through it is called electrical resistance.

The electronic theory explains the essence of the electrical resistance of metal conductors. Free electrons, when moving along a conductor, encounter atoms and other electrons on their way countless times and, interacting with them, inevitably lose part of their energy.

Electrons experience a kind of resistance to their movement. Different metal conductors, having different atomic structures, offer different resistance to electric current.

The same thing explains the resistance of liquid conductors and gases to the passage of electric current. However, we should not forget that in these substances it is not electrons, but charged particles of molecules that encounter resistance during their movement.

Resistance is denoted by the Latin letters R or r.

Ohm is the resistance of a column of mercury 106.3 cm high with a cross section of 1 mm 2 at a temperature of 0 ° C.

If, for example, the electrical resistance of a conductor is 4 ohms, then it is written like this: R = 4 ohms or r = 4 ohms.

To measure large resistances, a unit called megohm is used.

What kind of lighting do you prefer?

Built-in Chandelier

The greater the resistance of a conductor, the worse it conducts electric current, and, conversely, the lower the resistance of the conductor, the easier it is for electric current to pass through this conductor.

Consequently, to characterize a conductor (from the point of view of the passage of electric current through it), one can consider not only its resistance, but also the reciprocal of the resistance and called conductivity.

Electrical conductivity is the ability of a material to pass electric current through itself.

Since conductivity is the reciprocal of resistance, it is expressed as 1/ R, conductivity is denoted by the Latin letter g.

The influence of conductor material, its dimensions and ambient temperature on the value of electrical resistance

The resistance of various conductors depends on the material from which they are made. To characterize the electrical resistance of various materials, the concept of so-called resistivity has been introduced.

Specific resistance is the resistance of a conductor with a length of 1 m and a cross-sectional area of 1 mm 2. Resistivity is denoted by the letter p of the Greek alphabet. Each material from which a conductor is made has its own resistivity.

Expert opinion

It-Technology, Electrical power and electronics specialist

Ask questions to the “Specialist for modernization of energy generation systems”

Conductor cross-section as the most important criterion for choosing a wire In order to independently correctly calculate the cross-section or area of stranded wires, you must initially calculate the cross-section of each wire in the bundle, and then multiply the result by their total number. Ask, I'm in touch!

Cross-sectional area as an electrical quantity

The conductivity of the wire depends on the cross-section.
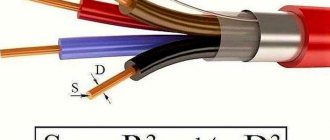
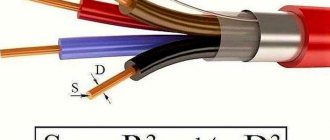
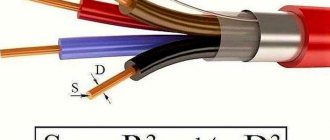
As an example of the cross-section, you can consider cutting the product at an angle of 90 degrees relative to the transverse axis. The outline of the resulting figure is determined by the configuration of the object. The cable looks like a small pipe, so when cut it will come out in the form of two circles of a certain thickness. When a round metal rod is cut transversely, the shape of a circle is obtained.

In electrical engineering, the PS area will mean the rectangular cross-section of the conductor in relation to its longitudinal part. The cross-section of the cores will always be round. The parameter is measured in mm2.

Novice electricians may confuse the diameter and cross-section of elements. To determine what cross-sectional area the core has, you need to take into account its round shape and use the formula:

S = πxR2, where:

S – area of the circle;
π—constant value 3.14;
R is the radius of the circle.

If the area indicator is known, it is easy to find the resistivity of the manufacturing material and the length of the wire. Next, the current resistance is calculated.

For convenience of calculations, the initial formula is transformed:

The radius is ½ the diameter.
To calculate area, π is multiplied by D (diameter) divided by 4, or 0.8 multiplied by 2 diameters.

When calculating, use the diameter indicator, since its incorrect selection can cause overheating and ignition of the cable.

Video

Electrical resistance
R
Dimension	L 2 MT −3 I −2 (SI); TL −1 (SGSE, Gaussian system); LT −1 (SGSM)
Units
SI	Ohm
SSSE	stat, s/cm
SGSM	abom, sm/s

Classical electrodynamics

Electricity Magnetism

Calculation goals

Cross-section of wires for lighting
It is necessary to calculate the parameters of the cross-sectional area of the conductor for several purposes:

obtaining the required amount of electricity to power household appliances;
eliminating overpayments for unused energy;
wiring safety and fire prevention;
the ability to connect high-power equipment to the network;
prevention of melting of the insulating layer and short circuits;
proper organization of the lighting system.

The optimal wire cross-section for lighting is 1.5 mm2 for the line, 4-6 mm2 for the input.

Ratio of cable diameter to cross-sectional area

Determining the cross-sectional area of conductors using a formula takes a long time. In some cases, it is appropriate to use data from a table. Since copper cable is used to organize modern wiring, the following parameters are entered into the table:

diameter;
cross-section in accordance with the diameter indicator;
maximum load power of conductors in networks with voltages of 220 and 380 V.

Core diameter, mm	Section parameters, mm2	Current strength, A	Copper conductor power, kW
Core diameter, mm	Section parameters, mm2	Current strength, A	Network 220 V	380 V network
1,12	1	14	3	5,3
1,38	1,5	15	3,3	5,7
1,59	2	19	4,1	7,2
1,78	2,5	21	4,6	7,9
2,26	4	27	5,9	10
2,76	6	34	7,7	12
3,57	10	50	11	19

By looking at the data in the appropriate columns, you can find out the necessary parameters for the power line of a residential building or industrial facility.

Steel circle weight table

The theoretical mass of 1 linear meter of a circle will be given in the table below. It will describe data on all cut diameters from 5 to 300 mm. And also the number of meters in a ton.

Circle diameter, mm	Meter weight, kg	Meters per ton
Circle 5	0.154	6493.51
Circle 5.5	0.187	5347.59
Circle 6	0.222	4504.5
Circle 6.3	0.245	4081.63
Circle 6.5	0.261	3831.42
Circle 7	0.302	3311.26
Circle 8	0.395	2531.65
Circle 9	0.499	2004.01
Circle 10	0.617	1620.75
Circle 11	0.746	1340.48
Circle 12	0.888	1126.13
Circle 13	1.042	959.69
Circle 14	1.208	827.81
Circle 15	1.387	720.98
Circle 16	1.578	633.71
Circle 17	1.782	561.17
Circle 18	1.998	500.5
Circle 19	2.226	449.24
Circle 20	2.466	405.52
Circle 21	2.719	367.78
Circle 22	2.984	335.12
Circle 23	3.262	306.56
Circle 24	3.551	281.61
Circle 25	3.853	259.54
Circle 26	4.168	239.92
Circle 27	4.495	222.47
Circle 28	4.834	206.87
Circle 29	5.185	192.86
Circle 30	5.549	180.21
Circle 31	5.925	168.78
Circle 32	6.313	158.4
Circle 33	6.714	148.94
Circle 34	7.127	140.31
Circle 35	7.553	132.4
Circle 36	7.990	125.16
Circle 37	8.440	118.48
Circle 38	8.903	112.32
Circle 39	9.378	106.63
Circle 40	9.865	101.37
Circle 41	10.364	96.49
Circle 42	10.876	91.95
Circle 43	11.400	87.72
Circle 44	11.936	83.78
Circle 45	12.485	80.1
Circle 46	13.046	76.65
Circle 47	13.619	73.43
Circle 48	14.205	70.4
Circle 50	15.413	64.88
Circle 52	16.671	59.98
Circle 53	17.319	57.74
Circle 54	17.978	55.62
Circle 55	18.650	53.62
Circle 56	19.335	51.72
Circle 58	20.740	48.22
Circle 60	22.195	45.06
Circle 62	23.700	42.19
Circle 63	24.470	40.87
Circle 65	26.049	38.39
Circle 67	27.676	36.13
Circle 68	28.509	35.08
Circle 70	30.210	33.1
Circle 72	31.961	31.29
Circle 73	32.855	30.44
Circle 75	34.680	28.84
Circle 78	37.510	26.66
Circle 80	39.458	25.34
Circle 82	41.456	24.12
Circle 85	44.545	22.45
Circle 87	46.666	21.43
Circle 90	49.940	20.02
Circle 92	52.184	19.16
Circle 95	55.643	17.97
Circle 97	58.010	17.24
Circle 100	61.654	16.22
Circle 105	67.973	14.71
Circle 110	74.601	13.4
Circle 115	81.537	12.26
Circle 120	88.781	11.26
Circle 125	96.334	10.38
Circle 130	104.195	9.6
Circle 135	112.364	8.9
Circle 140	120.841	8.28
Circle 145	129.627	7.71
Circle 150	138.721	7.21
Circle 155	148.123	6.75
Circle 160	157.834	6.34
Circle 165	167.852	5.96
Circle 170	178.179	5.61
Circle 175	188.815	5.3
Circle 180	199.758	5.01
Circle 185	211.010	4.74
Circle 190	222.570	4.49
Circle 195	234.438	4.27
Circle 200	246.615	4.05
Circle 210	271.893	3.68
Circle 220	298.404	3.35
Circle 230	326.148	3.07
Circle 240	355.126	2.82
Circle 250	385.336	2.6
Circle 260	416.779	2.4
Circle 270	449.456	2.22

Having the above table at hand, you will always know the exact weight of a steel circle of all diameters. And also their number of meters in one ton, which will not allow you to spend extra money when purchasing this rolled metal.

Source: domstrousam.ru

Calculation of the cross-section of a stranded conductor

Stranded wire consists of several individual wires. Its cross section is calculated as follows:

The indicator of the cross-sectional area of one core is found.
Cable cores are recalculated.
The quantity is multiplied by the cross-section of one core.

When connecting a stranded conductor, its ends are crimped with a special sleeve using crimping pliers.

Scattering of particle beams

In the frequently encountered case of elastic scattering of a beam of particles moving at the same speed at a certain center, the differential effective cross section

(dσdΩ{\displaystyle {d\sigma /d\Omega }}), characterizing the probability of scattering into a certain solid angle (dΩ{\displaystyle d\Omega }). It is equal to the ratio of the number of particles scattered per unit time per unit solid angle to the flux density of incident particles.

Integration over the full solid angle gives the total cross section

, for scattering at any angle:
σ=∫dσdΩdΩ{\displaystyle \sigma =\int {d\sigma \over d\Omega }d\Omega }
In the presence of inelastic interactions, the total cross section is the sum of the cross section for elastic and inelastic scattering. For each type (channel) of inelastic interactions, a separate effective cross section can be introduced.

Features of self-calculation

Independent calculation of the longitudinal section is performed on a core without an insulating coating. A piece of insulation can be moved or removed on a piece purchased specifically for testing. First you need to determine the diameter and find the cross section using it. Several methods are used for the work.

Using a caliper

The method is justified if the parameters of a truncated or defective cable are measured. For example, VVG may be designated as 3x2.5, but in fact be 3x21. Calculations are made as follows:

The insulating coating is removed from the conductor.
The diameter is measured with a caliper. You will need to place the wire between the legs of the instrument and look at the scale markings. The integer value is on top, the decimal value is on the bottom.
Based on the formula for finding the area of a circle S = π (D/2)2 or its simplified version S = 0.8 D², the cross section is determined.
The diameter is 1.78 mm. Substituting the value into the expression and rounding the result to hundredths, we get 2.79 mm2.

For domestic purposes, you will need conductors with a cross section of 0.75; 1.5; 2.5 and 4 mm2.

Using a ruler and pencil

Calculating PS using a ruler and pencil
If you do not have a special meter, you can use a pencil and ruler. Operations are performed with the test image:

An area of 5-10 cm is cleared of the insulating layer.
The resulting wire is wound around a pencil. Full turns are laid tightly, there should be no space between them, the “tails” are directed up or down.
Ultimately, a certain number of turns should be obtained; they need to be counted.
The winding is applied to the ruler so that the zero division coincides with the first winding.
The length of the segment is measured and divided by the number of turns. The resulting value is the diameter.
For example, it turned out 11 turns, which occupy 7.5 mm. When dividing 7.5 by 11, 0.68 mm is the cable diameter. The cross section can be found using the formula.

The accuracy of the calculations is determined by the density and length of the winding.

Table of correspondence between wire diameter and cross-sectional area

If it is not possible to test the diameter or make a calculation when purchasing, you can use a table. The data can be photographed, printed or transcribed, and then used to find the standard or popular core size.

Cable diameter, mm	Conductor cross-section, mm2
0,8	0,5
0,98	0,75
1,13	1
1,38	1,5
1,6	2
1,78	2,5
2,26	4
2,76	6
3,57	10

When purchasing an electrical cable, you will need to look at the parameters on the label. For example, VVNG 2x4 is used. The number of cores is the value after “x”. That is, the product consists of two elements with a cross section of 4 mm2. Based on the table, you can check the accuracy of the information.

Most often, the cable diameter is smaller than stated on the package. The user has two options - use a different one or choose a cable with a larger cross-sectional area in diameter. Having chosen the second one, you will need to check the insulation. If it is not solid, thin, or varies in thickness, choose products from another manufacturer.

Units and dimensions [edit | edit code]

Dimension of electrical resistance in the International System of Units: dim R

=
L
2
MT
−3
I
−2 . In the International System of Units (SI), based on the International System of Units, the unit of resistance is the ohm (Russian designation: Ом; international: Ω). In the SGS system as such, the unit of resistance does not have a special name, however, in its extensions (SGSE, SGSM and Gaussian system of units) the following are used [3]:

stat (in the SGSE and Gaussian system, 1 statΩ = (10 9 s
−2 ) s/cm = 898 755 178 736.818 Ohm (exactly) ≈ 8.98755 10 11 Ohm, equal to the resistance of the conductor through which a voltage of 1 statvolt flows current 1 statampere);
abom (in SGSM, 1 abΩ = 1·10 −9 Ohm = 1 nanoohm, equal to the resistance of the conductor through which a current of 1 abamp flows under a voltage of 1 abvolt).

The dimension of resistance in the SGSE and Gaussian system is equal to TL

−1 (that is, it coincides with the dimension of the inverse velocity, s/cm), in the SGSM -
LT
−1 (that is, it coincides with the dimension of velocity, cm/s) [4].

The reciprocal quantity in relation to resistance is electrical conductivity, the unit of measurement of which in the SI system is siemens (1 Sm = 1 Ohm −1), in the SGSE (and Gaussian) system it is staticsiemens, and in the SGSM it is absiemens [5].

Determination of the conductor cross-section at the input

You can check the nominal values in the Energosbyt company or in the documentation for the product. For example, the input rating of the machine is 25 A, the power consumption is 5 kW, the network is single-phase, 220 V.

The cross-section is selected so that the permissible current of the cores over a long period is greater than the rating of the machine. For example, a three-core copper conductor VVGng, laid in an open manner, was installed in a house. The optimal cross-section is 4 mm2, so you will need VVGng 3x4 material.

After this, the indicator of the conditional shutdown current for a machine with a nominal value of 25 A is calculated: 1.45x25 = 36.25 A. For a cable with a cross-sectional area of 4 mm2, the parameters of the continuous permissible current are 35 A, conditional - 36.25 A. In this case, it is better to take the input copper conductor with a cross-section of 6 mm2 and a permissible maximum current of 42 A.

Table of dependence of the current of the circuit breaker (fuse) on the cross-section

(Addition to article, June 2014)

And here is how the Germans treat the maximum current depending on the cross-sectional area of the wire. In the right column is a recommendation for choosing an automatic (protective) switch.

Table 3

Table for selecting a circuit breaker for different wire cross-sections

As you can see, the Germans are playing it safe and are providing for a larger reserve compared to us.

Although, perhaps this is because the table was taken from instructions from “strategic” industrial equipment.

Regarding the selection of wires, I usually use catalogs from online stores, here is an example of copper. They have the largest selection I've ever seen. It’s also good that everything is described in detail - composition, applications, etc.

A good Soviet book on the topic of the article:
• Karpov F. F. How to choose the cross-section of wires and cables, 1973 / Brochure from the Electrician's Library. Provides instructions and calculations necessary for selecting cross-sections of wires and cables up to 1000 V. Useful for those interested in primary sources., zip, 1.57 MB, downloaded: 4583 times./

Calculation of wire cross-section for a line of sockets

Cable cross-section for home electrical installations
Each electrical appliance has its own power ratings. They are measured in Watts and indicated in the passport or on a sticker on the case. An example of searching for a cross section would be a power line for a 2.4 kW washing machine. The calculations take into account:

wire material and installation method - three-core VVGng copper cable hidden in the wall;
cross-section features - the optimal value is 1.5 mm2, i.e. you will need a 3x1.5 cable;
using a socket. If only an automatic machine is connected, the characteristics will be sufficient;
The protection system is an automatic machine with a rated current of 10 A.

For double sockets, a copper cable with a cross-section of 2.5 mm2 and a 16 A circuit breaker are used.

Then data in meters or tons is entered into one of the fields

If you enter values in the "meters" field ("square meters" to find out the weight of the sheet), then you will know the total weight of the entire length (for example, the weight of the reinforcement).

If you are interested in calculating length by weight, then you need to enter data in the “tons” field.

Selection of cross-section for a three-phase 380 V line with several devices

Connecting several types of household appliances to a three-phase line involves the flow of consumed current through three wires. Each of them will have a smaller value than a two-core one. Based on this phenomenon, in a three-phase network it is permissible to use a cable with a smaller cross-section.

For example, a generator with a power of 20 kW and a total power of three phases of 52 A is installed in a house. Based on the table values, it turns out that the optimal cable cross-section is 8.4 mm2. Based on the formula, the actual cross-section is calculated: 8.4/1.75 = 4.8 mm2. To connect a 20 kW generator to a three-phase 380 V network, a copper conductor is required, the cross-section of each core is 4.8 mm2.

Metrological aspects [edit | edit code]

Instruments for measuring resistance [edit | edit code]

Ohmmeter
Measuring bridge
Ammeter and voltmeter (resistance is found using the formula)

Means of Reproduction of Resistance [edit | edit code]

Resistance store - set of resistors
Electrical resistance coils

State standard of resistance [edit | edit code]

GET 14-91 State primary standard of the unit of electrical resistance. Guardian Institute: VNIIM.

Wire cross-section in old buildings and maximum load

In Soviet-era high-rise buildings, aluminum wiring is used. Taking into account the correct connection of the nodes in the junction box, the quality of the insulation and the reliability of the connection contacts, it will last from 10 to 30 years.

If it is necessary to connect equipment with high energy consumption in houses with aluminum wiring, the cross-section and diameter of the cores are selected based on the power consumption. All data is shown in the table.

Current, A	Maximum power, VA	Cable diameter, mm	Cable cross-section, mm2
14	3000	1,6	2
16	3500	1,8	2,5
18	4000	2	3
21	4600	2,3	4
24	5300	2,5	5
26	5700	2,7	6
31	6800	3,2	8
38	8400	3,6	10

Weight of a steel circle. Table.

steel circles as blanks for making parts using mechanical processing. The circle is cut into blanks of the required length of the part, and then the turner produces the part we need on the machine. Also, steel circles are used in production (circles of small diameter), they are used in the manufacture of metal structures of varying complexity.

Round metal products are manufactured with a diameter of 5 to 300 millimeters; sizes larger than a diameter of 270 are manufactured at the discretion of the customer. According to the manufacturing method, they are distinguished: hot-rolled, calibrated (cold-rolled, cold-drawn) .

According to the degree of accuracy, round rolled metal can be: 1. High precision (indicated by the letter “A”), maximum deviations from the specified parameter will be from +0.3mm to -0.9mm; 2. Increased accuracy (indicated by the letter “B”), from +0.6mm to -2.0mm; 3. Normal accuracy (indicated by the letter “B”), from +0.8mm to -4.0mm.

The length of the rolled product depends on the quality of the steel, for example:

— rolled ordinary carbon and low-alloy steel is produced in the range from 2 to 12 meters;

— rolling of high-quality carbon and alloy steels — from 2 to 6 meters;

— low-alloy rolled products — from 1 to 6 meters.

Also, at the customer’s discretion, the circle can be made from 2 to 24 meters in length.

During production, they are guided by maximum deviations along the length of rolled products. There are three types of length deviations, namely: 1. up to 4 meters, 2. from 4 to 6 meters, 3. from 6 meters. In the first case, the value should not exceed 30 mm, in the second 50 mm, and in the third 70 mm. These deviations can be ignored at the discretion of the customer.