Most often, metals have crystal lattices of the following types: Body-centered cubic or abbreviated bcc (lead, tungsten) 9 atoms; Face-centered cubic (fcc) (silver, gold) 14 atoms; hexagonal close-packed (hcp) (magnesium, zinc). FCC and HCP lattices are more compact than bcc.
All metals are crystalline bodies that have a certain type of crystal lattice, consisting of low-mobility positively charged ions, between which free electrons move (the so-called electron gas
).
This type of structure is called a metallic bond
.
The type of lattice is determined by the shape of an elementary geometric body, the repeated repetition of which along three spatial axes forms the lattice of a given crystalline body.
Metals have relatively complex types of cubic lattices - body-centered (bcc) and face-centered (fcc) cubic lattices.
The basis of the bcc lattice is an elementary cubic cell (Fig. 1.2, b), in which positively charged metal ions are located at the vertices of the cube, and another atom in the center of its volume, i.e. at the intersection of its diagonals. Iron, chromium, vanadium, tungsten, molybdenum and other metals have this type of lattice in certain temperature ranges.
In an fcc lattice (Fig. 1.2, c), the unit cell is a cube with centered faces. Iron, aluminum, copper, nickel, lead and other metals have a similar lattice.
The third common type of close-packed lattices is hexagonal close-packed (HCP, Fig. 1.2, d). The GPU cell consists of spaces spaced from each other by a parameter c
parallel centered hexagonal bases. Three ions (atoms) are located on the middle plane between the bases.
For hexagonal lattices, the ratio of the parameter with
/
a
is always greater than one. Magnesium, zinc, cadmium, beryllium, titanium, etc. have such a lattice.
Didn't find what you were looking for? Use the search:
Best sayings:
Only sleep brings a student closer to the end of the lecture.
And someone else's snoring alienates him. 8588 – | 7405 – or read all.
91.146.8.87 © studopedia.ru Not the author of the materials posted. But it provides free use. Is there a copyright violation? Write to us | Feedback.
Disable adBlock! and refresh the page (F5)
very necessary
One of the most common materials that people have always preferred to work with has been metal. In each era, preference was given to different types of these amazing substances. Thus, the IV-III millennium BC is considered the Chalcolithic, or Copper Age. Later it is replaced by bronze, and then the one that is still relevant today comes into force - iron.
Today it is generally difficult to imagine that it was once possible to do without metal products, because almost everything, from household items, medical instruments to heavy and light equipment, consists of this material or includes individual parts from it. Why did metals manage to gain such popularity? Let’s try to figure out what the features are and how this is inherent in their structure.
General concept of metals
"Chemistry. 9th grade" is a textbook used by schoolchildren. It is here that metals are studied in detail. A large chapter is devoted to the consideration of their physical and chemical properties, because their diversity is extremely great.
Read also: The principle of operation of a car compressor
It is from this age that it is recommended to give children an idea of these atoms and their properties, because teenagers can already fully appreciate the significance of such knowledge. They see perfectly well that the variety of objects, machines and other things around them is based on a metallic nature.
What is metal? From the point of view of chemistry, these atoms are usually classified as those that have:
- a small number of electrons at the outer level;
- exhibit strong restorative properties;
- have a large atomic radius;
- As simple substances, they have a number of specific physical properties.
The basis of knowledge about these substances can be obtained by considering the atomic-crystalline structure of metals. It is this that explains all the features and properties of these compounds.
In the periodic table, most of the entire table is allocated to metals, because they form all the secondary subgroups and the main ones from the first to the third group. Therefore, their numerical superiority is obvious. The most common are:
All metals have a number of properties that allow them to be combined into one large group of substances. In turn, these properties are explained precisely by the crystalline structure of metals.
Educational materials
Of all the elements of the Periodic Table, D.I. Mendeleev 76 are metals. All metals have common characteristic properties that distinguish them from other substances. This is due to the peculiarities of their intra-atomic structure.
According to the modern theory of atomic structure, each atom represents a complex system, which can be schematically represented as consisting of a positively charged nucleus, around which negatively charged electrons move at different distances from it.
The attractive effect of the nucleus on the outer (valence) electrons in metals is largely compensated by the electrons of the inner shells. Therefore, valence electrons are easily detached and move freely between the resulting positively charged ions.
The weak connection of individual electrons with the rest of the atom is a characteristic feature of the atoms of metallic substances, which determines their chemical, physical and mechanical properties. The total number of electrons not associated with a particular atom in different metals is not the same. This explains the rather significant difference in the degree of “metallicity” of individual metals. The presence of electron gas also explains the special type of interatomic bonding inherent in metals.
The metallic type of bond is characterized by the fact that electrostatic attraction occurs between a lattice of positively charged ions and the free valence electrons surrounding them.
The presence of metallic bonds in metals gives them a number of characteristic properties: high thermal and electrical conductivity; increased ability to plastic deformation; thermionic emission, i.e. the ability to emit electrons when heated; good reflectivity, i.e. have a metallic luster and are opaque; positive temperature coefficient of electrical resistance, i.e. With increasing temperature, the electrical resistance of metals increases.
The last property is inherent only to metals, therefore:
A metal is a substance that has a metallic type of bond and a positive temperature coefficient of electrical resistance.
The bond strength in metals is determined by the relationship between the repulsive and attractive forces between ions and electrons. Atoms (ions) are located at such a distance from one another that the interaction energy is minimal (Fig. 1). Bringing atoms (ions) closer to a distance less than R0 or moving them away to a distance greater than R0 is only possible when a certain amount of work is performed against the forces of repulsion or attraction.
As a model of such interaction (Figure 1, b), we can take two balls (ions), between which there is a spring (interaction force). In equilibrium, the distance between the balls is R0. If the distance is reduced and the spring is compressed, a repulsive force (F) will appear between the balls, which will tend to return them to an equilibrium state. As the distance increases, a force of attraction (-F) will appear.
a b
Figure 1 — Forces of interaction between two atoms in a crystal lattice (a) and a model of such interaction (b)
In this regard, the atoms in metals are arranged regularly at a certain distance from each other, forming a regular crystal lattice.
It should be represented as straight lines mentally drawn in space in the direction of three coordinate axes, connecting the nearest atoms and passing through their centers, around which they perform oscillatory movements. The drawn lines form three-dimensional figures of regular geometric shape.
The smallest volume of a crystal that gives an idea of the atomic structure of the metal in its entire volume is called an elementary crystalline cell.
The dimensions of the crystal lattice are characterized by the parameters or lattice periods. The distance between the centers of neighboring atoms is measured in angstroms (1 Å=10-10 m), kiloixes (1 kX=1.00202 Å), nanometers
(1 nm=10-9 m). The lattice period of metals is in the range of 1…7 Å.
Half of the smallest distance between the centers of atoms is called the atomic radius.
The density of the crystal lattice, the volume occupied by atoms, is characterized by the coordination number.
The number of atoms located at an equal and smallest distance from a given atom is called the coordination number.
The higher the coordination number, the greater the atomic packing density. For a cubic cell, the coordination number is denoted by the letter “K”, and for a hexagonal cell - “G”.
The number of atoms per unit cell is called the basis.
The lattice basis is designated by the letter “n”. The main types of cells (Figure 2) that metals have are: body-centered cubic (bcc), face-centered cubic (fcc) and hexagonal close-packed (hcp).
There are two atoms (n=2) per unit cell of bcc: one is in the center of the cube, and the other is contributed by atoms located at the vertices of the cube. Each atom at the vertex of the cube simultaneously belongs to eight conjugated unit cells, and a given cell accounts for only 1/8, and for the entire cell (1/8) x 8 = 1 atom. The coordination number is calculated for the central atom and corresponds to K8.
There are four atoms per fcc unit cell (n=4): one of them is contributed by atoms at the vertices of the cube, and three are contributed by atoms located in the middle of the face, since each of these atoms belongs simultaneously to two cells: (1/2) x6=3. The coordination number is calculated for the atom located in the center of the face and corresponds to K12.
There are six atoms (n=6) per unit cell of the hcp: three of them are located inside the cell. Two atoms in the center of the faces give one atom: (1/2)x2=1, and twelve vertex atoms give two atoms: (1/6)x12=2. The coordination number is calculated for the atom located inside the cell and corresponds to G12.
Polymorphic transformations > Continue >
Properties of metals
The specific properties of the substances in question include the following.
- Metallic shine. All representatives of simple substances have it, and most have the same silvery-white color. Only a few (gold, copper, alloys) are different.
- Malleability and plasticity - the ability to deform and recover quite easily. It is expressed to different degrees in different representatives.
- Electrical and thermal conductivity are one of the main properties that determine the areas of application of a metal and its alloys.
The crystalline structure of metals and alloys explains the reason for each of the indicated properties and speaks about their severity in each specific representative. If you know the features of such a structure, then you can influence the properties of the sample and adjust it to the desired parameters, which is what people have been doing for many decades.
Crystallization of alloys
The transition of a metal from a liquid to a solid state with the formation of a crystalline structure is called primary crystallization.
The formation of new crystals in a crystalline solid is called secondary crystallization (recrystallization).
The crystallization process consists of two simultaneous processes:
- crystal nucleation;
- linear crystal growth;
Crystals can nucleate spontaneously (spontaneous crystallization) or nucleate and grow on existing ready-made crystallization centers (non-spontaneous crystallization) (Figure 33).
Figure 34 Growth of nucleation centers and crystal growth
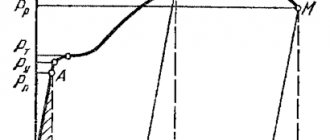
Spontaneous crystallization (Fig. 35) is due to the desire of the substance to have a more stable state, characterized by a decrease in the thermodynamic potential G, a characteristic of the free energy of the system. The second law of thermodynamics is that any system always strives to occupy a state such that it has min free energy. The temperature at which the thermodynamic potentials of a substance, both in solid and liquid states, are equal is called the equilibrium temperature (thermodynamic temperature) TG.
Fig.35 Spontaneous crystallization
Thermodynamic potential is determined by:
G = E – TS + РV (according to Helmholtz)
where G is the thermodynamic potential, the free energy of the system,
E – internal energy of the system,
T – thermodynamic temperature
S – entropy (state function: order and disorder associated with translational and oscillatory motion),
РV – work of external forces (pressure on volume)
G = H – TS (according to Gibbs)
where H is enthalpy (E + РV) the sum of the work of internal and external forces.
The difference between the equilibrium ( ТG .) and real ( Тр ) crystallization temperature is called the degree of supercooling ( ΔТ ).
The formation of nuclei is facilitated by fluctuations , i.e. deviation of the energy of groups of atoms in individual zones of a liquid metal from a certain average value.
The appearance of nuclei changes the thermodynamic potential (free energy) of the entire system. On the one hand, during the transition of a liquid to a crystalline state, the thermodynamic potential G decreases, on the other hand, it increases (+) due to the appearance of an interface between the liquid and the crystalline nucleus.
Figure 36 shows how the free energy of the system changes during crystallization.
Kinetics of crystallization . The rate of formation of nuclei formed per unit time per unit volume (1mm-3s-1); growth rate - an increase in the linear dimensions of the growing crystal per unit time (mm/s). Both processes are associated with the movement of atoms and depend on temperature (degree of supercooling Δ T).
Non-spontaneous crystallization (heterogeneous)
Under real conditions, crystallization processes and the nature of the forming structures largely depend on the available ready-made crystallization centers. These centers are:
- refractory particles of non-metallic inclusions;
- oxides;
- intermetallic compounds formed by impurities.
Refining the structure helps improve the mechanical properties of the metal.
Fig. 36 Change in free energy during crystallization
In practice, a technological operation called modification . It consists of introducing modifiers (boron into steel, sodium into aluminum and its alloys). Cooling the metal before pouring to temperatures slightly above the melting point of the metal helps to reduce the grain size.
Crystal Formation
The shape and size of grains formed during crystallization depend on:
- speed and direction of heat removal:
- temperature of liquid metal;
- impurity content.
The structure of the ingot depends on many factors: (Fig. 37)
- quantity and properties of impurities in pure metal;
- the amount of alloying elements in the alloy;
- alloy casting temperature;
- cooling rate during crystallization, etc.
Fig. 37 Diagram of the structure of a metal ingot obtained at different temperatures
The typical structure of an alloy ingot consists of three zones: (Fig. 38)
- small equiaxed crystals on the surface of the ingot, due to the high degree of supercooling;
- columnar crystals, most favorably oriented with respect to heat removal, located normal to the walls of the mold;
- equiaxed large crystals in the middle of the ingot, where the lowest degree of supercooling is observed and no directional heat removal is felt.
A structure consisting of only columnar crystals is called transcrystalline. Found in ingots of very pure metals.
Chemical heterogeneity in individual zones of the ingot is called zonal segregation . It negatively affects the mechanical properties of the alloy. In real alloys, in addition to zonal segregation, there are other types of segregation.
Atomic crystal structure of metals
What is this structure, what is it characterized by? The name itself suggests that all metals are crystals in the solid state, that is, under normal conditions (except for mercury, which is a liquid). What is a crystal?
This is a conventional graphic image constructed by intersecting imaginary lines through the atoms that line up the body. In other words, every metal is made up of atoms. They are located in it not chaotically, but very correctly and consistently. So, if you mentally combine all these particles into one structure, you will get a beautiful image in the form of a regular geometric body of some shape.
This is what is commonly called the crystal lattice of a metal. It is very complex and spatially voluminous, therefore, for simplicity, not all of it is shown, but only a part, an elementary cell. A set of such cells, collected together and reflected in three-dimensional space, forms crystal lattices. Chemistry, physics and metallurgy are sciences that study the structural features of such structures.
The unit cell itself is a set of atoms that are located at a certain distance from each other and coordinate a strictly fixed number of other particles around themselves. It is characterized by packing density, distance between constituent structures, and coordination number. In general, all these parameters are characteristics of the entire crystal, and therefore reflect the properties exhibited by the metal.
Read also: Do-it-yourself scraper for walk-behind tractor
There are several types of crystal lattices. They all have one feature in common: the nodes contain atoms, and inside there is a cloud of electron gas, which is formed by the free movement of electrons inside the crystal.
Structure and state of aggregation of substances
There are three states of aggregation: solid, liquid and gas. Each of them assumes a certain arrangement of particles. Below we will describe in more detail how the crystal lattice and the aggregative state of a substance are related in chemistry, but for now we will highlight the general principles.
- If the particles move chaotically, and the distance between them is many times greater than their own sizes, it is a gas . Due to the great distance from each other, the molecules and atoms in such a substance weakly interact with each other.
- If the particles are still arranged randomly, but at a small distance from each other, it is a liquid . In the liquid state of a substance, its molecules and atoms have stronger bonds that are more difficult to break.
- If the particles are collected close to each other and in a certain order, it is a solid . In this state, the connections between them are strongest. Particles can only move within their location and hardly move in space.
Most substances can be in a solid, liquid, or gaseous state, and depending on pressure and temperature, they can easily change from one to another. A typical example is water, which turns into steam when heated and becomes solid ice when cooled.
Types of crystal lattices
Fourteen lattice structure options are usually combined into three main types. They are as follows:
- Body-centered cubic.
- Hexagonal close-packed.
- Face-centered cubic.
The crystal structure of metals was studied only through electron microscopy, when it became possible to obtain high magnification images. And the classification of types of lattices was first given by the French scientist Bravais, by whose name they are sometimes called.
Body-centered lattice
The structure of the crystal lattice of metals of this type is the following structure. This is a cube with eight atoms at its nodes. Another one is located in the center of the free internal space of the cell, which explains the name “body-centered”.
This is one of the options for the simplest structure of the unit cell, and therefore the entire lattice as a whole. The following metals have this type:
- molybdenum;
- vanadium;
- chromium;
- manganese;
- alpha iron;
- beta iron and others.
The main properties of such representatives are a high degree of malleability and ductility, hardness and strength.
What are pearlite and eutectoid
Observations show that this transition occurs as follows: upon reaching temperatures GS along the boundaries Observations show that this transition occurs as follows: upon reaching temperatures GS along the boundaries of austenite crystals, the first portions of α - Fe, i.e., ferrite, are released, the amount of which gradually increases.
Since ferrite almost does not dissolve carbon, during the transition γ-Fe -> α-Fe, the carbon concentration in retained austenite gradually increases and can be determined by the GS line depending on temperature. The ferrite separation process continues until the carbon concentration corresponds to point 5, i.e., up to C = 0.83%, and the temperature reaches t = 723°.
At point S, the GS curve intersects with ES, the curve of the limiting solubility of carbon in austenite. Therefore, further saturation of retained austenite with carbon becomes impossible, and subsequent cooling causes the final decomposition of austenite, which occurs at a constant temperature t = 723°.
During this decomposition, the γ-Fe->α-Fe transition is completed, and the carbon released from the iron crystal lattice forms F3C cementite particles. The decomposition of austenite occurs in a confined space within each grain, so the decomposition products (ferrite and cementite) are formed in the form of closely mixed particles, usually in the form of alternating plates of ferrite and cementite.
Scheme of changes in the structure of steels when passing through critical points
This decomposition product of austenite is called pearlite; Since pearlite has a structure similar to eutectic, it is called a eutectoid. The difference between eutectic and eutectoid is that eutectic is formed from a liquid solution, while eutectoid is formed from a solid.
The formation of pearlite begins and ends at a constant t=723°. This is how the ferrite-pearlite structure of steels appears, which, upon further cooling from t = 723°, no longer undergoes any structural changes. The figure shows the microstructures of pure iron and steel at C = 0.15% and at C = 0.6% (magnification 100) after etching the scratched surface of a microsection with a 4% solution of HNO3 in ethyl alcohol.
Rice. 1. - ferrite in pure iron. Rice. 2 Hypoeutectoid steel with C content = 0.15%
In Fig. 1, which shows the microstructure of pure iron, the boundaries between the light ferrite grains are clearly visible. In Fig. Figure 2 shows the microstructure of building steel (C=0.15%); light fields are ferrite, dark areas are pearlite. In Fig. Figure 3 shows the microstructure of engineering steel (C = 0.6%), from which axles, shafts, connecting rods, etc. are made; Most of the section is occupied by pearlite, and ferrite is observed only in the form of a thin mesh. The more carbon, the more pearlite in the steel structure; the composition of pearlite is the same (C = 0.83%). The structure of perlite is usually lamellar (Fig. 4).
Rice. 3 Hypoeutectoid steel with C content = 0.6%. Rice. 4 Eutectoid steel (lamellar pearlite).
Ferrite, as mentioned above, is the softest plastic component of iron-carbon alloys; cementite, which is part of pearlite, is the hardest and most brittle, therefore, with increasing carbon content, the strength and hardness of steel increases, but ductility and toughness decrease
In order for construction steel to be sufficiently ductile, the amount of perlite in it should not exceed 25%, which corresponds to a carbon content of up to 0.2%.
In those parts that require greater strength and hardness, but lower ductility and toughness are acceptable (machine parts), steels with a large amount of pearlite, with a C content of up to 0.6%, are used. In the construction industry, such steels are used, for example, for the manufacture of shovels and supporting parts of bridge trusses.
Face-centered lattice
The crystal structure of metals having a face-centered cubic lattice is the following structure. This is a cube that includes fourteen atoms. Eight of them form lattice nodes, and another six are located, one on each face.
They have a similar structure:
The main distinctive properties are shine of different colors, lightness, strength, malleability, increased resistance to corrosion.
Iron, properties of the atom, chemical and physical properties.
Fe 26 Iron
55.845(2) 1s2 2s2 2p6 3s2 3p6 3d6 4s2
Iron is an element of the periodic system of chemical elements of D.I. Mendeleev with atomic number 26. It is located in the 8th group (according to the old classification - a secondary subgroup of the eighth group), the fourth period of the periodic system.
Iron atom and molecule. Iron formula. Structure of the iron atom
Isotopes and modifications of iron
Properties of iron (table): temperature, density, pressure, etc.
Physical properties of iron
Chemical properties of iron. Interaction of iron. Chemical reactions with iron
Getting iron
Application of iron
Table of chemical elements D.I. Mendeleev
Defects in the crystal structure of metals
However, all types of cells considered may also have natural shortcomings, or so-called defects. This may be due to various reasons: foreign atoms and impurities in metals, external influences, and so on.
Therefore, there is a classification that reflects the defects that crystal lattices may have. Chemistry as a science studies each of them in order to identify the cause and method of elimination so that the properties of the material are not changed. So, the defects are as follows.
- Spot. They come in three main types: vacancies, impurities or dislocated atoms. They lead to deterioration of the magnetic properties of the metal, its electrical and thermal conductivity.
- Linear or dislocation. There are edge and screw ones. They deteriorate the strength and quality of the material.
- Surface defects. Affects the appearance and structure of metals.
Currently, methods have been developed to eliminate defects and obtain pure crystals. However, it is not possible to completely eradicate them; an ideal crystal lattice does not exist.
General structure
Metals are solid substances with a crystalline structure. The exception is mercury, a liquid metal. Crystal lattices are metal atoms ordered in a certain way. Each atom consists of a positively charged nucleus and several negatively charged electrons. Metal atoms don't have enough electrons, so they are ions.
A unit of a crystal lattice is an elementary crystal cell, in the conventional nodes and on the faces of which there are positively charged ions. They are held together by metallic bonds that arise due to the random movement of electrons separated from the atoms (due to which the atoms turned into ions).
Negatively charged electrons keep positively charged electrons at an equal distance, giving the crystal lattice the correct geometric shape. Rice. 1. Metal connection diagram.
The free movement of electrons determines the electrical and thermal conductivity of metals.
The importance of knowledge about the crystalline structure of metals
From the above material, it is obvious that knowledge about the fine structure and structure makes it possible to predict the properties of the material and influence them. And the science of chemistry allows you to do this. The 9th grade of a general education school places emphasis in the learning process on developing in students a clear understanding of the importance of the fundamental logical chain: composition - structure - properties - application.
Read also: Automotive voltage regulator circuit
Information about the crystalline structure of metals very clearly illustrates this dependence and allows the teacher to clearly explain and show children how important it is to know the fine structure in order to correctly and competently use all the properties.
Content:
Definition of crystal lattice
As we know, all material substances can exist in three basic states: liquid, solid, and gaseous. True, there is also a state of plasma, which scientists consider no less than the fourth state of matter, but our article is not about plasma. The solid state of a substance is therefore solid because it has a special crystalline structure, the particles of which are in a certain and clearly defined order, thus creating a crystal lattice. The structure of the crystal lattice consists of repeating identical elementary cells: atoms, molecules, ions, and other elementary particles connected by various nodes.
Types of crystal lattices
Depending on the particles of the crystal lattice, there are fourteen types of it, here are the most popular of them:
- Ionic crystal lattice.
- Atomic crystal lattice.
- Molecular crystal lattice.
- Metal crystal lattice.
Next, we will describe in more detail all types of crystal lattice.
Main types of metal crystal lattices
To share, click
Main types of metal crystal lattices
The physical properties of metals are determined by their electronic structure and the nature of the crystal lattice.
Because metallic bonding is unsaturable and nondirectional, metals are characterized by crystalline structures with high coordination numbers (the number of atoms that surround one atom). As a rule, metals crystallize in one of three types of lattices, for two of them the coordination number is 12, and for the third - 8.
The structure of the first two crystal lattices can be represented as follows. Let's mentally place the spherical metal atoms on the table and move them tightly towards each other. We will notice that each ball will be surrounded by six neighboring balls (a in the figure below).
Then put the second layer (B) on top of the first layer of balls (A) so that the balls of the second layer fall into the recesses between the balls of the first layer (b in the figure above). Let's do the same thing, placing the balls of the third layer on top. Laying the third layer balls is possible in two different ways. However, this does not affect the coordination number, but results in two unequal structures.
The first method leads to the location of the balls of the third layer exactly above the balls of the first layer (c in the figure above). This structure is called hexagonal close packing (hcp) and consists of alternating layers of ABAB metal atoms. The word hexagonal means hexagonal and indicates that each ball in its layer is surrounded by a hexagon of its nearest neighbors.
The second method differs from the first in that the location of the balls of the third layer C is rotated 60 degrees around the vertical axis with respect to the first layer (d in the figure above). In this case, the balls of the third layer appear above the recesses between the balls of the first layer. In this case, only the next, fourth, layer of balls exactly repeats the structure of the balls of the first layer. This structure is called cubic close packing (CPU) or face-centered cubic packing (FCC) and consists of alternating layers of ABCAB metal atoms.
The third crystal structure (coordination number equal) can be considered as the center of a cube, at the vertices of which are its eight nearest neighbors. This structure is called body-centered cubic packing (BCC) .
Unit cells for all types of metal crystal lattices are shown in the figure below.
The described crystal structures differ in the degree of space filling: the most densely packed (the degree of space filling is 74%) are the crystalline lattices of the GPU and KPU, the less densely packed (68%) is the OCU type lattice. Voids play an important role in determining the structure of metal compounds and their alloys.
Crystal lattices of some metals
| Grate type | Metal |
| GPU | Mg, Zn, Be, Cd, Os, Ru |
| KPU (GCC) | Cu, Ag, Al, Ca, Ni, Au, Pb, Pd, Pt, Co, Sr |
| OCU | Li, Na, K, Rb, Cs, V, Cr, Mo, W, Fe, Ba |
It is also important to know that a number of metals, depending on temperature, can crystallize in different types of crystal lattices (the phenomenon of polymorphism ), for example, white and gray tin.
Atomic crystal lattice
Substances with an atomic crystal lattice, as a rule, have strong covalent bonds in their nodes consisting of atoms themselves. A covalent bond occurs when two identical atoms share fraternal electrons with each other, thus forming a common pair of electrons for neighboring atoms. Because of this, covalent bonds bind atoms tightly and evenly in a strict order - perhaps this is the most characteristic feature of the structure of the atomic crystal lattice. Chemical elements with similar bonds can boast of their hardness and high melting point. Chemical elements such as diamond, silicon, germanium, and boron have an atomic crystal lattice.
Crystal structure of materials
In nature, there are two types of solids that differ in their properties : crystalline and amorphous (Fig. 1).
Rice. 1 Crystal structure of matter
When cooling amorphous bodies, there is no obvious transition temperature from the liquid to the solid state, while crystalline bodies have a specific crystallization temperature (Fig. 2).
Rice. 1. 2 Cooling curves: 1- amorphous bodies; 2 – crystalline
When heated, amorphous
Crystalline bodies remain solid, i.e. retain their given shape until a very specific temperature, at which they turn into a liquid state. Crystalline bodies are characterized by an ordered arrangement in space of the elementary particles (ions, atoms, molecules) from which they are composed - the geometric factor (Fig. 3)
Fig.3 Crystal lattices
The properties of crystals depend on:
- on the electronic structure of atoms.
- the nature of their interaction in the crystal.
- on the spatial arrangement of elementary particles.
- chemical composition.
- size and shape of crystals.
Depending on the size of the structural components and the methods used to identify them, the following concepts are used:
- fine structure. (x 20000 – 150000)
- microstructure. (up to x1500) Fig. 4, a
- macrostructure. (up to x10) Fig. 4, b
a b
Fig.4 Microstructure (a) and macrostructure (b) of the alloy
Crystal cell . In a crystal, the elementary particles (ions, atoms, molecules) from which the crystal is built are brought together until they touch and are located differently, but naturally in different directions. The smallest parallelepiped in which elementary particles are located at the nodes is called an elementary cell. Its sequential movement forms a spatial crystal lattice.
To describe the unit cell of a crystal lattice, six quantities are used: three segments - lattice period a, b, c, three angles between these segments α, β, γ (Fig. 5)
The relationships between these quantities determine the shape of the cell. Based on the shape of the unit cells, all crystals are divided into seven systems (Fig. 6)
In most cases, lattices are complex, since elementary particles are located not only at the nodes of the crystal lattice, but also on its edges or in the center of the lattice (Fig. 7, 8, 9)
The degree of complexity is judged by the number of particles per unit cell. In a simple spatial lattice there is always one particle per cell. There are two particles per bcc There are four particles per fcc
The system, period, and number of particles per unit cell completely determine the location of elementary particles in the crystal.
Fig.5 Space-crystalline lattice
Crystal systems of elements
| System | Period | Angles |
| Triclinic | a ≠ b ≠ c | α ≠ β ≠ γ |
| Monoclinic | a ≠ b ≠ c | α = β = 90о γ ≠ 90о |
| Rhombic | a ≠ b ≠ c | α = β = γ =90о |
| Rhombohedral | a = b = c | α = β = γ ≠ 90о |
| Hexagonal | a = b ≠ c | α = β = 90о γ = 120о |
| Tetragonal | a = b ≠ c | α = β = γ =90о |
| Cubic | a = b = c | α = β = γ =90о |
Fig. 6 Seven unit cell systems
Fig 7 Body-centered cubic (BCC) lattice
Fig.8 Face-centered cubic (fcc) lattice
Fig.9 Hexagonal close-packed lattice (hcp)
Additional characteristics: coordination number and compactness coefficient.
Coordination number is the number of nearest and equidistant elementary particles. ( TO ). For BCC – K8 . For HCC – K12 . For a simple cubic lattice - K6 .
The compactness coefficient is the ratio of the volume of all elementary particles per elementary cell to the entire volume of the elementary cell.
For a simple cubic lattice – 0.52. For bcc – 0.68. For HCC – 0.74.
The remaining space is formed by pores, which are differentiated into octahedral (octahedral) and tetrahedral (tetrahedral).
Crystallographic indices.
The properties in parallel directions are the same, so it is enough to indicate for the entire family of parallel lines one direction passing through the origin of coordinates (a crystal lattice node). The coordinates of this node are expressed as integers u , v, w in units of segments a , b, c, enclosed in square brackets [ u, v, w] and called direction indices. They are always expressed as integers, and a negative value is indicated by a minus sign above the subscript.
The position of the plane in space is determined by the segments cut off by the plane along the x , y, z axes. These segments are expressed as integers m , n, p in units of segments a , b, c. It is customary to take reciprocal segments as plane indices: h = 1/m; k = 1/n; l = 1/p. These three numbers (h, k, l) enclosed in parentheses are called plane indices. If the plane cuts off negative segments along the axes, this is marked with a minus sign above the corresponding index (Fig. 10).
Anisotropy.
(Greek Anises unequal + tropes properties)
This is the dependence of the properties of a crystal on direction, resulting from the ordered arrangement of atoms (ions, molecules) in space. In a crystal, the distances between atoms in different crystallographic directions are different, and therefore the properties are different. The strength and ductility of a single crystal varies depending on the direction. Under natural conditions, crystalline bodies are polycrystals, i.e. consist of many small differently oriented crystals. In this regard, polycrystalline bodies are considered to be pseudo-isotropic. During pressure treatment of a polycrystal, crystallographic planes of the same index in different grains can be oriented in parallel. Such polycrystals are called textured , and they, like single crystals, are anisotropic (Fig. 11).
Fig. 10 Crystallographic indices
Rice. 11 Change in structure during plastic deformation
The influence of the type of bond on the structure and properties of crystals.
The type of connection that occurs between elementary particles in a crystal is determined by the electronic structure of the atoms that interact. Elementary particles in a crystal come closer to a certain distance, which is determined by the interaction of forces acting in the crystal. Attractive forces arise due to the interaction of electrons with the positively charged nucleus of their own atom, as well as with the positively charged nuclei of neighboring atoms. Repulsive forces arise as a result of the interaction of positively charged nuclei of neighboring atoms as they approach each other. They appear at close proximity and grow more intensely than the forces of attraction.
Balancing of forces occurs when atoms approach each other at a distance d o . This approach corresponds to the maximum binding energy Eb, which makes the crystal thermodynamically stable (Fig. 12).
Fig. 12 Resultant force and binding energy in crystals
Ecv determines: melting temperature, evaporation temperature, elastic modulus, temperature coefficient of linear expansion.
All crystals are divided according to the nature of the prevailing bond:
- molecular;
- covalent;
- metal;
- ionic.
Molecular crystals.
In crystals of inert gases, the van der Waals bond is the only one, and, therefore, it determines the structure and properties of the crystals.
Covalent crystals.
A covalent bond is characterized by directionality, since each atom enters into an exchange interaction with a certain number of neighboring atoms.
Due to the high binding energy, covalent crystals are characterized by high melting and evaporation temperatures.
Metal crystals.
In a metal crystal, when interacting with elements of other groups, atoms easily give up their valence electrons and turn into positive ions. The metallic bond is non-directional, since each atom strives to attract as many neighboring atoms as possible.
Among metals and some non-metals, the phenomenon of polymorphism - the ability in the solid state at different temperatures (or pressure) to have different types of crystal structures of bcc, fcc, hcp , etc. These crystal structures are called allotropic forms or modifications. The low-temperature modification is called α , and the high-temperature modification is β, γ, δ, etc.
The stability of modifications at a certain temperature and pressure is determined by the value of the thermodynamic potential (free energy G).
G = H – ST
More stable at a given temperature will be a modification that has a lower algebraic value of the thermodynamic potential, which can be achieved either due to low enthalpy H or high entropy S.
Enthalpy H – (Greek: enthalpy heat) is a physical function of independent variables – pressure and entropy, which uniquely determines the state of the phases. Systems in thermodynamics (thermodynamic potential).
Entropy S (Greek ep in, inward + trope rotation, transformation) is a measure of the internal disorder of a system.
About thirty metals exhibit temperature polymorphism Rapid cooling can preserve a high-temperature modification for a long time at T = 20-25°C , since the low diffusion mobility of atoms at such temperatures is not capable of causing lattice rearrangement. In addition, polymorphism under the influence of temperature and pressure (graphite - diamond) is known.
Ionic crystals.
In complex crystals consisting of elements of different valencies, the formation of an ionic type of bond is possible. A representative of this group is the FeO oxide crystal, the lattice of which consists of negatively charged oxygen ions and positively charged iron ions. The redistribution of valence electrons during an ionic bond occurs between the atoms of one molecule (one iron atom and one oxygen atom).
Crystal defects.
The structure of real crystals differs from ideal ones. Real crystals always contain defects, and therefore there is no ideally correct arrangement of atoms throughout the entire volume of the crystal.
Crystal defects are divided into:
- point;
- linear;
- superficial;
Point defects. These include: vacancies, interstitial atoms of the main substance, foreign interstitial atoms (Fig. 13).
Vacancies are the most important type of point defects; they accelerate all processes associated with the movement of atoms: diffusion, sintering of powders, etc. In technically pure metals, point defects increase electrical resistance, but have almost no effect on mechanical properties.
Linear defects are edge and screw dislocations (Fig. 14). An edge dislocation in cross-section represents the edge of “extra” half-plane in the lattice. Around dislocations the lattice is elastically distorted. The measure of distortion is the so-called Burgers vector (Fig. 15). For an edge dislocation, the Burgers vector is equal to the interatomic distance and is perpendicular to the dislocation line; for a screw dislocation, it is parallel to it.
In Fig. Figure 16 shows a diagram of the movement of dislocations under the action of tangential stresses (τ).
Figure 17 shows the dependence of the deformation resistance of metals, determined by the values of Ϭв, Ϭт, on the dislocation density (ρ), which is defined as their total length, cm, in 1 cm3, i.e. expressed in cm-2. The theoretically calculated strength of an ideal crystal is 103 times higher than the experimentally determined values for a real crystal. The strength of practically defect-free crystals—whiskers—approaches the theoretical strength. To date, it has been possible to obtain crystals that are practically free of dislocations. These whiskers are small in size (2–10 mm long and 0.5–2.0 μm thick) and have strength close to theoretical. Thus, filamentary iron crystals have a tensile strength Ϭв = 13000 MPa, and technical iron - only 300 MPa. Pure and annealed metals have the lowest strength values (dislocation density 106 – 108 cm-2).
The scientists' calculations showed that the highest strength that can be achieved by increasing the number of defects in the metal is about 1010 - 1012 cm2. This is explained by the fact that dislocations begin to interfere with deformation displacements of each other (the formation of dislocation networks).
If the number of dislocations exceeds 1012 - 1013, then the strength of the metal drops sharply due to the destruction of crystals in places where there is a large accumulation of defects.
Fig. 13 Vacancy and interstitial atom
Currently, there are many ways to increase the strength of products (alloying, metal forming, heat treatment, etc.), but we still cannot say exactly what strength is. There cannot be a clear answer to this question, however. On the one hand, strength is resistance to plastic deformation; on the other hand, resistance to brittle fracture; third, wear resistance; fourth, resistance to corrosion; with the fifth, the ability to withstand high temperatures under load, etc.
Depending on the conditions under which a particular part must work, certain qualities attached to it turn out to be more important. That is why, when speaking further about the strength of metal products, each time we will mean not some abstract strength, but the very specific ability of a metal product to resist certain external influences.
A
b
Fig. 14 Edge dislocation (a) and screw dislocation (b)
A
b
Fig. 15 Contour and Burgers vector at an edge (a) and screw (b) dislocation
Fig. 16 Schemes of dislocation motion
Surface defects. The most important surface defects are high-angle and low-angle boundaries, stacking faults, and twin boundaries (Fig. 18, 19).
The boundaries between grains are called more angular, since the corresponding crystallographic directions in neighboring grains form angles of tens of degrees. Each grain, in turn, consists of subgrains and blocks.
A subgrain is a part of a crystal with a relatively regular structure. Subgrain boundaries are walls of dislocations that separate the grain into individual subgrains and blocks. The angle of mutual misorientation between neighboring subgrains is small (no more than 5°), therefore such boundaries are called low-angle. Impurities also accumulate at low-angle boundaries.
Fig. 17 Dependence of deformation resistance on the number of defects
Fig. 18 Subgrain boundary
Fig. 19 Boundaries of grains and subgrains
A stacking fault is a part of the atomic plane limited by dislocations, within which the normal order of alternation of atomic layers is disrupted (Fig. 20).
Fig.20 Packaging defect
Twin boundaries represent the formation in a single crystal of regions with a regularly changed orientation of the crystal structure. The structures of twin formations are either a mirror image of the atomic structure of the parent crystal (matrix) in a certain plane, or are formed by rotating the matrix structure around the crystallographic axis by a certain angle, constant for a given substance, or by other symmetry transformations. The pair - matrix and twin formation - is called a twin, Fig. 21.
Fig. 21 Boundary of twins
Conclusions.
Surface defects affect the mechanical and physical properties of materials. Grain boundaries are especially important. The yield strength σt is related to the grain size d by the dependence σt = σо + k d-1/2 , where σо and k are constants for a given material. The finer the grain, the higher the yield strength, viscosity and the lower the risk of brittle fracture. The size of subgrains has a similar, but weaker effect on the mechanical properties.
The grain size is determined according to GOST (Fig. 22).
Phase composition of alloys.
In alloys, elements can interact with each other in different ways, forming crystalline phases that differ in chemical composition, type of bond, and structure. These crystals, depending on their atomic crystal structure, are usually divided into two main types:
- solid solutions;
- intermediate phases
Solid solutions are crystals in which the crystal lattice of one element of the solvent is preserved.
Fig.22 Grain size scale, X 100 (GOST 5639-82)
Such solutions are crystalline phases of variable composition. They can be of unlimited solubility for substitution solid solutions and limited solubility for substitution and interstitial solid solutions.
Substitutional solid solutions (Fig. 23).
Interstitial solid solutions (Fig. 24). Such solid solutions arise when transition metals fuse with nonmetals having a small atomic radius - H, N, C, B.
Interstitial solid solutions always have limited solubility and occur mainly when the solvent has an hcp or fcc lattice.
Conclusion : Solid solutions form the basis of most industrial structural alloys and special purpose alloys. They are distinguished by good technological ductility: they are well deformed in a hot state, and many in a cold state.
Fig. 23 Substitutional solid solutions
Intermediate phases.
The intermediate phase is called crystals formed by various elements and having their own type of crystal lattice , different from the lattices of their constituent elements.
Intermediate phases are designated in the same way as solid solutions, by letters of the Greek alphabet.
These phases also include chemical compounds (Fig. 25). They have the following features:
- composition, which can be expressed by the simple formula AnBm, where A and B are the corresponding elements, and n and m are integers;
- crystal lattice , different from the crystal lattices of the elements forming the compounds;
- properties that differ sharply from the properties of the elements forming the compound;
- constant crystallization temperature , like that of pure metals.
Fig. 24 Interstitial solid solutions
Fig. 25 Crystal lattice of cementite (iron carbide)
Diffusion in metals and alloys.
Diffusion is the transfer of dissimilar atoms, which is accompanied by a change in the concentration of components in individual zones of the alloy.
Self-diffusion is the transition of metal atoms from a crystal lattice site to an adjacent or intersite under the influence of thermal excitation.
Mechanisms of diffusion (self-diffusion) (Fig. 26)
Fig. 26 Diffusion in metals
Liquid crystals
Liquid crystals are liquids with an ordered molecular structure (mainly organic substances in which the molecules have an elongated shape).
Liquid crystals are fluid, like ordinary liquids, but at the same time they have anisotropy of properties, like crystals (Fig. 27)
During the transition, due to increased thermal vibrations, the ordered molecular structure completely disappears, the transparency of the substance increases , and therefore the upper temperature point of the existence of a liquid crystal is often called the clearing point .
Based on their structure, liquid crystals are divided into three classes:
- nematic - molecules are arranged in chains;
- smectic - molecules form parallel layers;
- cholesteric - molecules are arranged in a spatial spiral.
Liquid crystals are used to make medical thermometers to monitor overheating of components and parts, and converters of invisible infrared radiation into visible light. In the latter case, absorption of infrared radiation heats the liquid crystal so that the color of the reflected light changes. Liquid crystals are used in modulators, information display systems - calculators, wrist watches, automobile measuring instruments, devices for deflecting light flux, etc.
Cholesteric phase
Fig.27 Liquid crystal textures
Structure of polymers, glass and ceramics.
Polymers are substances with a large molecular weight, whose molecules consist of identical groups of atoms - units (monomer) (Fig. 28, 29). Depending on the nature of the bonds between linear molecules, polymers are divided:
- thermoplastic;
- thermosetting.
Thermoplastic polymers are capable of repeatedly softening when heated and hardening when cooling without changing their properties.
Thermosetting polymers remain solid when heated until complete thermal decomposition.
Polymers based on macromolecule structure.
Fig. 28 Structure of polymers
Ceramics are materials obtained by high-temperature sintering of mineral powders (Fig. 30). A characteristic feature of ceramic materials is fragility.
Sitalls or glass-crystalline materials are obtained from glasses of a special composition using controlled crystallization.
Photositalls are used as photosensitive materials.
Thermositalls have universal applications: as wear-resistant materials they are used for parts of hydraulic machines, friction units, and protective enamels; as strong, stable dielectrics for radio components, circuit boards, etc. (Fig. 31).
Glass is an amorphous substance formed by the fusion of oxides or without oxide compounds. The basis of the glass is formed by a volumetric mesh of homogeneous structural elements. The structure of amorphous glass appears when the glass mass is cooled, when an increase in its viscosity prevents crystallization (Fig. 32).
Fig. 29 Production of products from thermoplastic polymers
Fig.30 Ceramic parts
Fig.31 Parts made of glass-crystalline materials
Fig.32 Glass furniture
Metal crystal lattice
The type of bond of a metal crystal lattice is more flexible and ductile than the ionic one, although in appearance they are very similar. Its distinctive feature is the presence of positively charged cations (metal ions) at lattice sites. Between the nodes live electrons that participate in the creation of the electric field; these electrons are also called electric gas. The presence of such a structure of a metal crystal lattice explains its properties: mechanical strength, heat and electrical conductivity, fusibility.